Jan Dangerfield, Stuart Haring and, Julian Gilbey Solutions for Exercise 2: EXERCISE 4A

A gardener is trying to move a heavy roller of mass $150 \mathrm{kg}$ along rough ground at an angle of $5°$ to the horizontal. He exerts a force of $200 \mathrm{N}$ down the slope and parallel to it and the roller is on the point of slipping.

What assumptions have been made to answer the question?

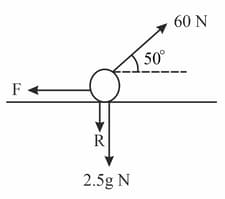
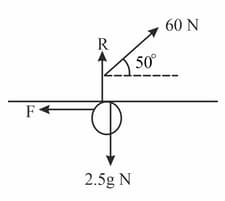
A ring of mass $2.5 \mathrm{kg}$ is threaded onto a fixed horizontal wire. It is made of a rubbery material to give it an extremely high coefficient of friction and prevent it from sliding along the wire. When it is at rest, the higher part of the ring is in contact with the wire, so the normal contact force from the wire is upwards, as shown in the diagram. The ring is attached to a string, which provides a tension of $60 \mathrm{N}$ at an angle of $50°$ above the horizontal. The ring is now in limiting equilibrium. The force diagram for the situation is given in the diagram. Note that the normal contact force is now acting downwards because there cannot be a vertical component of acceleration, so the lower part of the ring is now in contact with the wire. Find the coefficient of friction between the ring and the wire.

A box of mass $50 \mathrm{kg}$ is at rest on a slope, which is at an angle of $26°$ to the horizontal. The coefficient of friction is $0.4.$ The box is held in place by a rope attached to a winch pulling up the slope and parallel to it. Find the minimum and maximum possible values for the tension, $T,$ which the winch could provide for the box to remain in equilibrium.

A car of mass $1350 \mathrm{kg}$ is at rest on a rough slope at an angle of $7°$ to the horizontal. A man tries to push it down the slope, exerting a force of $500 \mathrm{N},$ but cannot get it to move.

Find the angle that the total contact force makes with the slope.

A car of mass $1350 \mathrm{kg}$ is at rest on a rough slope at an angle of $7°$ to the horizontal. A man tries to push it down the slope, exerting a force of $500 \mathrm{N},$ but cannot get it to move.

When the man stops pushing, the car remains in equilibrium. Find the angle that the total contact force makes with the slope.

A ring of mass $2 \mathrm{kg}$ is held in place at rest on a rough horizontal wire. It is attached to a string that is at an angle of $40°$ above the horizontal.

Explain why once the ring is released it can never be in equilibrium, however high the coefficient of friction, when the tension in the string satisfies $T\sin 40=2\mathrm{g}.$

A ring of mass $2 \mathrm{kg}$ is held in place at rest on a rough horizontal wire. It is attached to a string that is at an angle of $40°$ above the horizontal.

Show that when the tension is $100 \mathrm{N}$ the coefficient of friction must be at least $1.73$ for the ring to be in equilibrium, but when the tension increases to $200 \mathrm{N}$ the coefficient of friction can be as low as $1.41$ with the ring remaining in equilibrium. Explain why.

A ring of mass $3 \mathrm{kg}$ is at rest on a rough horizontal wire. It is attached to a string that is at an angle of $60°$ above the horizontal. The coefficient of friction between the ring and the wire is $0.7.$ Find the set of values for the tension, $T,$ which will allow the ring to remain in equilibrium.