M L Aggarwal Solutions for Exercise 4: Chapter Test

In the given figure, a chord $PQ$ of a circle with centre $O$ and radius $15$cm is bisected at $M$ by a diameter $AB$. If $OM=9$cm, find the lengths of:-

$\left(\mathrm{i}\right) PQ$

$\left(\mathrm{ii}\right) AP$

$\left(\mathrm{iii}\right) BP$

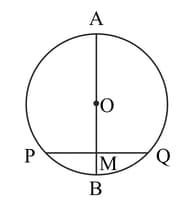
The radii of two concentric circles are $17$cm and $10$ cm; a line$PQRS$ cuts the larger circle at $P$ and $S$ and the smaller circle at $Q$ and $R$ . If $QR=12$ cm, calculate $PQ$.

A chord of length $48$ cm is at a distance of $10$cm from the centre of a circle. If another chord of length $20$cm is drawn in the same circle, find its distance from the centre of the circle.

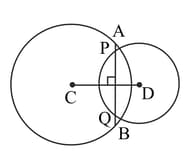
In the figure $\left(\mathrm{i}\right)$given below, two circles with centres $C, D$ intersect in points $P, Q.$ if length of common chord is $6$cm and $CP=5$cm, $DP=4$cm, calculate the distance $CD$ correct to two decimal places.

In the figure $\left(\mathrm{ii}\right)$given below, $P$ is a point of intersection of two circles with centres $C$ and $D$. if the straight line $APB$ is parallel to $CD$, prove that $AB=2CD.$

In the figure given below $C$ and $D$ are centres of two intersecting circles. The line $APQB$ is perpendicular to the line of centres $CD.$ Prove that

$\left(\mathrm{i}\right) AP=QB \left(\mathrm{ii}\right) AQ=BP$

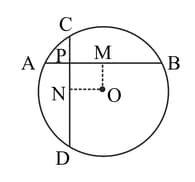
In the figure given below two equal chords $AB$ and $CD$ of a circle with centre $O$ intersect at right angles at $P.$ If $M$ and $N$ are mid-points of the chords $AB$ and $CD$ respectively, prove that $NOMP$ is a square.

In the given figure, $AD$ is a diameter of a circle. If the chord $AB$ and $AC$ are equidistant form its centre $O$, prove that $AD$ bisects $\angle BAC$ and $\angle BDC$.