R. D. Sharma Solutions for Chapter: Surface Areas and Volumes, Exercise 4: REVISION EXERCISE

A solid metallic sphere of diameter $28 \mathrm{cm}$ is melted and recast into a number of smaller cones, each of diameter $4\frac{2}{3} \mathrm{cm}$ and height $3 \mathrm{cm}$. Find the number of cones so formed.

A wall $24 \mathrm{m}, 0.4 \mathrm{m}$ thick and $6 \mathrm{m}$ high is constructed with the bricks each of dimensions $25 \mathrm{cm}\times 16 \mathrm{cm}\times 10 \mathrm{cm}$. If the mortar occupies $\frac{1}{10} \mathrm{th}$ of the volume of the wall, then find the number of bricks used in constructing the wall.

A bucket is in the form of a frustum of a cone and holds $28.490$ liters of water. The radii of the top and bottom are $28 \mathrm{cm}$ and $21 \mathrm{cm}$, respectively. If the height of the bucket is $x \mathrm{cm}$, find the value of $x$. (Round off the value of $x$ to the nearest integer.)

Marbles of diameter $1.4 \mathrm{cm}$ are dropped into a cylindrical beaker of diameter $7 \mathrm{cm}$ containing some water. Find the number of marbles that should be dropped into the beaker so that the water level rises by $5.6 \mathrm{cm}$.

Two cones with same base radius $8 \mathrm{cm}$ and height $15 \mathrm{cm}$ are joined together along their bases. If the surface area of the shape formed is $k {\mathrm{cm}}^2$, then find the value of $k$.  $(\text{Take} \mathrm{\pi }=22/7)$

From a solid cube of side $7 \mathrm{cm}$, a conical cavity of height $7 \mathrm{cm}$ and radius $3 \mathrm{cm}$ is hollowed out. If the volume of the remaining solid is $k {\mathrm{cm}}^3$, then find the value of $k$.  $\left(\text{Take} \mathrm{\pi }=22/7\right)$

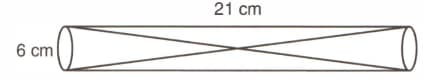
Two solid cones $A$ and $B$ are placed in a cylindrical tube as shown in the figure below. The ratio of their capacities are $2:1$. Find the heights and capacities of the cones. If the volume of the remaining portion of the cylinder is $\mathrm{k} {\mathrm{cm}}^3$ then find $\mathrm{k}$. $\left(\text{Take} \mathrm{\pi }=22/7\right)$

An ice-cream cone with hemispherical top is full of ice-cream having radius $5 \mathrm{cm}$ and height $10 \mathrm{cm}$. If the volume of ice-cream, provided that its $\frac{1}{6}$ part is left unfilled with ice-cream is $k {\mathrm{cm}}^3$, then find the value of $k$, correct up to two decimal places.  $\left(\text{Take} \mathrm{\pi }=22/7\right)$