Sue Pemberton, Julianne Hughes and, Julian Gilbey Solutions for Chapter: Vectors, Exercise 4: EXERCISE 9B

The diagram shows a cuboid $OABCDEFG$ with a horizontal base $OABC.$ The cuboid has a length $OA$ of $8 \mathrm{cm},$ a width $AB$ of $4 \mathrm{cm}$ and a height $BF$ of $d \mathrm{cm}.$ The point $M$ is the midpoint of $CE$ and the point $N$ is the point on $GF$ such that$GN=6 \mathrm{cm}.$ The unit vectors $i, j$ and $k$ are parallel to $OA, OC$ and $OD,$ respectively. It is given that $\overrightarrow{OM}=4i+2j+k.$

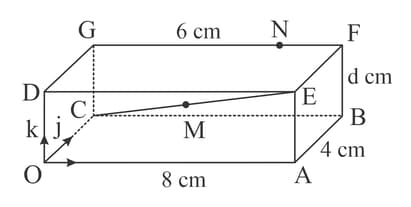
Write down the height of the cuboid. (Enter the answer excluding units)

The diagram shows a triangular prism, $OABCDE.$ The uniform cross-section of the prism, $OAE,$ is a right-angled triangle with base $24 \mathrm{cm}$ and height $7 \mathrm{cm}.$ The length, $AB,$ of the prism is $20 \mathrm{cm}.$ The unit vectors $i, j$ and $k$ are parallel to $\overrightarrow{OA},\overrightarrow{ OC}$ and $\overrightarrow{OE,}$ respectively. The point $N$ divides the length of the line $DB$ in the ratio $2 : 3.$

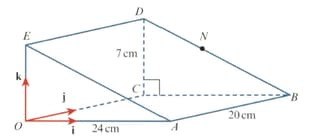
Find the magnitude of $\overrightarrow{DB}$. (write the answer without units).

The diagram shows a triangular prism, $OABCDE.$ The uniform cross-section of the prism, $OAE,$ is a right-angled triangle with base $24 \mathrm{cm}$ and height $7 \mathrm{cm}.$ The length, $AB,$ of the prism is $20 \mathrm{cm}.$ The unit vectors $i, j$ and $k$ are parallel to $\overrightarrow{OA},\overrightarrow{ OC}$ and $\overrightarrow{OE,}$ respectively. The point $N$ divides the length of the line $DB$ in the ratio $2 : 3.$

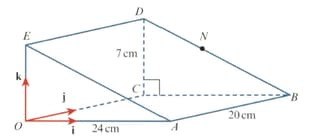
Find $\overrightarrow{ON}.$

Three points $O, P$ and $Q$ are such that $\overrightarrow{OP}=2\hat{i}+5\hat{j}+a\hat{k}$ and $\overrightarrow{OQ}=\hat{i}+\left(1+a\right)\hat{j}-3\hat{k}$. Given that the magnitude of $\overrightarrow{OP}$ is equal to the magnitude of $\overrightarrow{OQ},$ find the value of the constant $a.$

Relative to an origin $O,$ the position vectors of the points $P$ and$Q$ are given by $\overrightarrow{OP}=\left(\begin{array}{c}-6k\\ -2\\ 8(1+k)\end{array}\right)$ and $\overrightarrow{OQ}=\left(\begin{array}{c}2k+13\\ -8\\ -32k\end{array}\right).$

Given that $OPQ$ is a straight line find the value of the constant $k$.

Relative to an origin $O,$ the position vectors of the points $P$ and$Q$ are given by $\overrightarrow{OP}=\left(\begin{array}{c}-6k\\ -2\\ 8(1+k)\end{array}\right)$ and $\overrightarrow{OQ}=\left(\begin{array}{c}2k+13\\ -8\\ -32k\end{array}\right).$

Given that $OPQ$ is a straight line:

write each of $\overrightarrow{OP}$ and $\overrightarrow{OQ}$ in the form $xi+yj+zk$

Relative to an origin $O,$ the position vectors of the points $P$ and$Q$ are given by $\overrightarrow{OP}=\left(\begin{array}{c}-6k\\ -2\\ 8(1+k)\end{array}\right)$ and $\overrightarrow{OQ}=\left(\begin{array}{c}2k+13\\ -8\\ -32k\end{array}\right).$

Given that $OPQ$ is a straight line:

If the magnitude of the vector $\overrightarrow{PQ}$ is $3\sqrt{n}$, then the value of $n$ is

An ant has an in-built vector navigation system! It is able to go on complex journeys to find food and then return directly home (to its starting point) by the shortest route.
An ant leaves home and its journey is represented by the following list of displacements:

$i+3j+6k \left(\begin{array}{l}1\\ 6\\ 0\end{array}\right) 10i \left(\begin{array}{c}-3\\ -1\\ -5\end{array}\right) 4i-k$

The ant finds food at this point.

What single displacement takes the ant home?

One unit of displacement is $10 \mathrm{cm}.$

What is the shortest distance home from the point where the ant finds food?