Using Ampere’s circuital law, obtain the expression for the magnetic field due to a long solenoid at a point inside the solenoid on its axis.

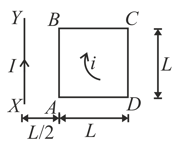
In a thin rectangular metallic strip a constant current I flows along the positive x - direction, as shown in the figure. The length, width and thickness of the strip are l, w and d, respectively. A uniform magnetic field $\overrightarrow{\text{B}}$ is applied on the strip along positive y - direction. Due to this the charge carries experience a net deflection along the z-direction. This results in accumulation of charge carriers on the surface PQRS and appearance of equal opposite charges on the face opposite to PQRS. A potential difference along the z - direction is thus developed. Charge accumulation continues until the magnetic force is balanced by the electric force. The current is assumed to be uniformly distributed on the cross section of the strip and carried by electrons.

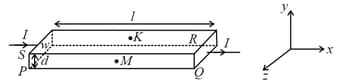
Consider two different metallic strips (1 and 2) of the same material. Their lengths are the same, widths are $w_1$ and $w_2$ and thicknesses are $d_1$ and $d_2$ , respectively. Two points $K$ and $M$ are symmetrically located on the opposite faces parallel to the $x$ - $y$ plane (see figure). $V_1$ and $V_2$ are the potential differences between $K$ and $M$ in strips 1 and 2 respectively. Then, for a given current $I$ flowing through them in a given magnetic field strength $B$ , the correct statement(s) is (are)

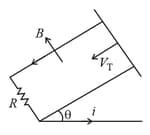
A copper rod of mass $m$ slides under gravity on two smooth parallel rails $l$ distance apart and set an angle $\theta$ to the horizontal. At the bottom, the rails are joined by a resistance $R$ in figure. There is a uniform magnetic field $B$ perpendicular to the plane of the rails. The terminal velocity of rod is

A solenoid of $1000$ turns per metre has a core with relative permeability $500.$ Insulated windings of the solenoid carry an electric current of $5 \mathrm{A}.$ The magnetic flux density produced by the solenoid is:

(Permeability of free space$=4\pi \times {10}^{-7} \mathrm{H} {\mathrm{m}}^{-1}$)

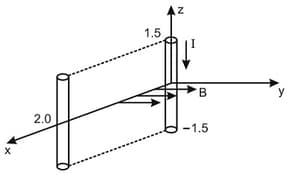
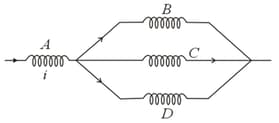
Four identical long solenoids $\mathrm{A}, \mathrm{B}, \mathrm{C}$ and $\mathrm{D}$ are connected to each other as shown in the figure. If the magnetic field at the center of $\mathrm{A}$ is $3 \mathrm{T}$ the field at the center of $\mathrm{C}$ would be : (Assume that the magnetic field is confined with in the volume of respective solenoid).

Choose the correct statement in the following:

(a) The magnetic field inside the solenoid is greater than that of outside
(b) The magnetic field inside an ideal solenoid is not at all uniform
(c) The magnetic field at the centre, inside an ideal solenoid is almost twice that at the ends
(d) The magnetic field at the centre, inside an ideal solenoid is almost half of that at the ends

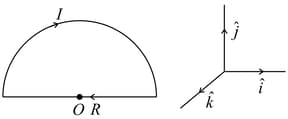
The magnitude and direction of a force vector acting on a unit length of thin wire carrying a current $I$ at point $O$, if the wire has a semicircular shape of radius $R$ as shown in the figure.

In a thin rectangular metallic strip a constant current I flows along the positive x - direction, as shown in the figure. The length, width and thickness of the strip are l, w and d, respectively. A uniform magnetic field $\overrightarrow{\text{B}}$ is applied on the strip along positive y - direction. Due to this the charge carries experience a net deflection along the z-direction. This results in accumulation of charge carriers on the surface PQRS and appearance of equal opposite charges on the face opposite to PQRS. A potential difference along the z - direction is thus developed. Charge accumulation continues until the magnetic force is balanced by the electric force. The current is assumed to be uniformly distributed on the cross section of the strip and carried by electrons.

Consider two different metallic strips (1 and 2) of same dimensions (length $l$ , width $w$ and thickness $d$ ) with carrier densities $n_1$ and $n_2$ , respectively. Strip 1 is placed in magnetic field $B_1$ and strip 2 is placed in magnetic field $B_2$ , both along positive $y$ -directions. Then $V_1$ and $V_2$ are the potential differences developed between $K$ and $M$ in strips 1 and 2 respectively. Assuming that the current $I$ is the same for both strips, the correct option(s) is (are)