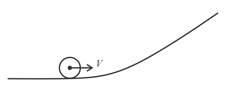
A hollow sphere is rolling (without slipping) on horizontal surface with velocity $v$. It then moves up a curved track. If sliding does not occur, then the maximum height moved-up by sphere is

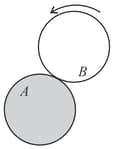
A flat surface of a thin uniform disk $A$ of radius $R$ is glued to a horizontal table. Another thin uniform disk $B$ of mass $M$ and with the same radius $R$ rolls without slipping on the circumference of $A$, as shown in the figure. A flat surface of $B$ also lies on the plane of the table. The center of mass of $B$ has fixed angular speed $\omega$ about the vertical axis passing through the center of $A$. The angular momentum of $B$ is $nM\omega R^2$ with respect to the center of $A$. Which of the following is the value of $n$?

​​​​​​​

A disc of mass $M$ and radius $R$ rolls without slipping on a horizontal surface (see figure).

If the speed of its centre is $v_0$, then the magnitude of the angular momentum of the disc about a fixed point $P$ at a height $5\mathrm{R}/2$ above the horizontal surface

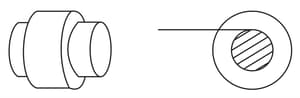
At time $t=0$, a disk of radius $1 \mathrm{m}$ starts to roll without slipping on a horizontal plane with an angular acceleration of $\alpha =\frac{2}{3} \mathrm{rad} {\mathrm{s}}^{-2}$. A small stone is stuck to the disk. At $t=0$, it is at the contact point of the disk and the plane. Later, at time $t=\sqrt{\pi } \mathrm{s}$, the stone detaches itself and flies off tangentially from the disk. The maximum height (in $\mathrm{m}$) reached by the stone measured from the plane is $\frac{1}{2}+\frac{x}{10}$. The value of $x$ is [Take $g=10 \mathrm{m} {\mathrm{s}}^{-2}$.]

If the numerical value has more than two decimal places, truncate/round-off the value to TWO decimal places.

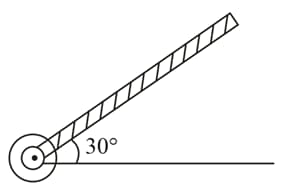
A rod of length $50 cm$ is pivoted at one end. It is raised such that it makes an angle of ${30}^o$ from the horizontal as shown and released from rest. Its angular speed when it passes through the horizontal (in $rad s^{-1}$ ) will be $\left(g=10 m{s}^{-2}\right)$

A small roller of diameter $20 \mathrm{cm}$ has an axle of diameter $10 \mathrm{cm}$ (see figure below on the left). It is on a horizontal floor and a meter scale is positioned horizontally on its axle with one edge of the scale on top of the axle (see figure on the right). The scale is now pushed slowly on the axle so that it moves without slipping on the axle, and the roller starts rolling without slipping. After the roller has moved $50 \mathrm{cm},$ the position of the scale will look like (figures are schematic and not drawn to scale)

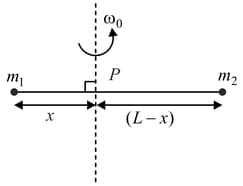
Point masses ${\mathrm{m}}_1$ and ${\mathrm{m}}_2$ are placed at the opposite ends of rigid rod of length $\text{L}$, and negligible mass. The rod is to be set rotating about an axis perpendicular to it. The position of point $\text{L}$ on this rod through which the axis should pass so that the work required to set the rod rotating with angular velocity ${\mathrm{\omega }}_0$ is minimum, is given by:

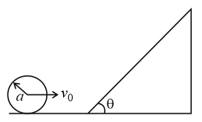
A sphere of radius $a$ and mass $m$ rolls along a horizontal plane with constant speed $v_0$. It encounters an inclined plane at angle $\theta$ and climbs upward. Assuming that it rolls without slipping, how far up the sphere will travel?