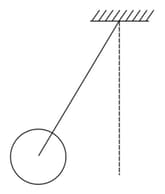
A metal rod of length $L$ and mass $m$ is pivoted at one end. A thin disk of mass $M$ and radius $R$ ($<L$) is attached at its centre to the free end of the rod. Consider two ways the disc is attached:

(Case $A$): The disc is not free to rotate about its centre and

(Case $B$): the disc is free to rotate about its centre.

The rod- disc system performs SHM in vertical plane after being released from the same displaced position. Which of the following statement(s) is (are) true?

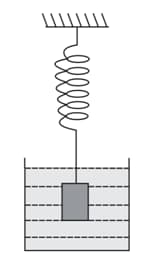
Consider the spring-mass system with the mass submerged in water as shown in the figure. The phase space diagram for one cycle of this system is,

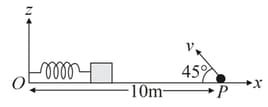
A small block is connected to one end of a massless spring of un-stretched length $4.9 \mathrm{m}$. The other end of the spring (see the figure) is fixed. The system lies on a horizontal frictionless surface. The block is stretched by $0.2 \mathrm{m}$ and released from rest at $t=0$. It then executes simple harmonic motion with angular frequency, $\mathrm{\omega }=\frac{\mathrm{\pi }}{3} \mathrm{rad} {\mathrm{s}}^{-1}$. Simultaneously, at $t=0$, a small pebble is projected with speed $v$ from point $P$ at an angle of $45°$ as shown in the figure. The point $P$ is at a horizontal distance of $10 \mathrm{cm}$ from $O$. If the pebble hits the block at $t=1 \mathrm{s}$, the value of $v$ is (take, $g=10 \mathrm{m} {\mathrm{s}}^{-2}$),

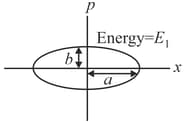
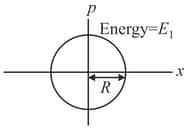
Two independent harmonic oscillators of equal masses oscillate about the origin with angular frequencies ${\mathrm{\omega }}_1$ and ${\mathrm{\omega }}_2$ and have total energies $E_1$ and $E_2$, respectively. The variations of their momenta $p$ with positions $x$ are shown in figures. If $\frac{a}{b}=n^2$ and $\frac{a}{R}=n$, then the correct equation(s) is (are),

A block with mass $\mathrm{M}$ is connected by a massless spring with stiffness constant $\mathrm{k}$ to a rigid wall and moves without friction on a horizontal surface. The block oscillates with small amplitude $\mathrm{A}$ about an equilibrium position${\mathrm{x}}_0$. Consider two cases :

$\left(\mathrm{i}\right)$when the block is at ${\mathrm{x}}_0$ ; and

$\left(\mathrm{ii}\right)$ when the block is at $\mathrm{x} = {\mathrm{x}}_0 + \mathrm{A}.$In both the cases, a particle with mass $\mathrm{m} (< \mathrm{M})$ is softly placed on the block after which they stick to each other.

Which of the following statement(s) is(are) true about the motion after the mass $\mathrm{m}$ is placed on the mass $\mathrm{M}$?

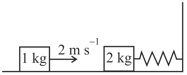
A spring-block system is resting on a frictionless floor as shown in the figure. The spring constant is $2.0 \mathrm{N} {\mathrm{m}}^{-1}$ and the mass of the block is$2.0 \mathrm{kg}$. Ignore the mass of the spring. Initially, the spring is in an un-stretched condition. Another block of mass $1.0 \mathrm{kg}$ moving with a speed of $2.0 \mathrm{m} {\mathrm{s}}^{–1}$ collides elastically with the first block. The collision is such that the $2.0 \mathrm{kg}$ block does not hit the wall. The distance, in metres, between the two blocks when the spring returns to its un-stretched position for the first time after the collision is ____.