A particle with total mechanical energy which is small and negative is under the influence of a one dimensional potential U x = x 4 4 - x 2 2 J , where x is in meters. At time t = 0 s , it is at x = - 0 . 5 m . Then at a later time it can be found,

between x = - 1 . 0 m to x = 0 . 0 m

between x = - 1 . 0 m to x = 1 . 0 m

between x = 0 . 0 m to x = 1 . 0 m

EASY

Earn 100

A particle moves along a curve of unknown shape but magnitude of force F is constant and always acts along tangent to the curve. Then

50% studentsanswered this correctly

Important Questions on Work, Energy and Power

MEDIUM

Physics>Mechanics>Work, Energy and Power>Work and Power

An asteroid of mass

m

is approaching earth, initially at a distance

10 R_{e}

with speed

v_{i} .

It hits Earth with a speed

v_{f},

(R_{e}

and

M_{e}

are radius and mass of earth

)

, then

EASY

Physics>Mechanics>Work, Energy and Power>Work and Power

Blocks of masses

m, 2 m, 4 m

and

8 m

are arranged in a line of a frictionless floor. Another block of mass

m

, moving with speed

υ

along the same line (see figure) collides with mass

m

in perfectly inelastic manner. All the subsequent collisions are also perfectly inelastic. By the time the last block of mass

8 m

starts moving the total energy loss is

p %

of the original energy. Value of

p

is close to:
Question Image

MEDIUM

Physics>Mechanics>Work, Energy and Power>Work and Power

A mass of $0.5 kg$ moving with a speed of $1.5 m s^{- 1}$ on a horizontal smooth surface, collides with a nearly weightless spring of force constant $k = 50 N m^{- 1}$ . The maximum compression of the spring would be

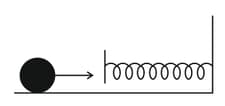
Question Image

EASY

Physics>Mechanics>Work, Energy and Power>Work and Power

A block of mass

100 g

moving at a speed of

2 m s^{- 1}

compresses a spring through a distance

2 cm

before its speed is halved. Find the spring constant of the spring

MEDIUM

Physics>Mechanics>Work, Energy and Power>Work and Power

A particle with total mechanical energy which is small and negative is under the influence of a one dimensional potential

U (x) = \frac{x^{4}}{4} - \frac{x^{2}}{2} J

, where

x

is in meters. At time

t = 0 s

, it is at

x = - 0.5 m

. Then at a later time it can be found,

MEDIUM

Physics>Mechanics>Work, Energy and Power>Work and Power

A uniform chain of mass

M

and length

L

is lying on a smooth horizontal table, with half of its length hanging down. The work done in pulling the entire chain up the table is

MEDIUM

Physics>Mechanics>Work, Energy and Power>Work and Power

If the potential energy between two molecules is given by

U = \frac{A}{r^{6}} - \frac{B}{r^{12}},

then at equilibrium, separation between molecules, and the potential energy are:

EASY

Physics>Mechanics>Work, Energy and Power>Work and Power

A body is released from a height of

30 m

vertically downwards. The speed of the body at which potential energy is twice that of kinetic energy is (Acceleration due to gravity

= 10 m s^{- 2}

)

EASY

Physics>Mechanics>Work, Energy and Power>Work and Power

Two particles

A

and

B

of same mass have their de-Broglie wavelengths in the ratio

X_{A} : X_{B} = K : 1 .

Their potential energies

U_{A} : U_{B} = 1 : K^{2} .

The ratio of their total energies

E_{A} : E_{B}

MEDIUM

Physics>Mechanics>Work, Energy and Power>Work and Power

A person trying to lose weight by burning fat lifts a mass of 10 kg upto a height of 1m 1000 times. Assume that the potential energy lost each time he lowers the mass is dissipated. How much fat will he use up considering the work done only when the weight is lifted up? Fat supplies

3.8 \times 10^{7}

J of energy per kg which is converted to mechanical energy with a 20% efficiency rate. Take

g = 9.8 m s^{- 2}

EASY

Physics>Mechanics>Work, Energy and Power>Work and Power

If a spring of spring constant

200 N m^{- 1}

is compressed by

5 cm

, then the energy stored in the spring is

HARD

Physics>Mechanics>Work, Energy and Power>Work and Power

A particle is moving in a circle of radius

r

under the action of a force

F = α r^{2}

which is directed towards centre of the circle. Total mechanical energy (kinetic energy + potential energy) of the particle is (take potential energy

= 0

for

r = 0

MEDIUM

Physics>Mechanics>Work, Energy and Power>Work and Power

The potential energy function for a two dimensional force is given by

U = 3 x^{3} y - 7 x

. The force that acts at the point

(x, y)

is (Take

\hat{i}

and

\hat{j}

as unit vectors along

X

- and

Y

- axes)

MEDIUM

Physics>Mechanics>Work, Energy and Power>Work and Power

A body is moving unidirectionally under the influence of a source of constant power. The square of its displacement in time

t

is proportional to

HARD

Physics>Mechanics>Work, Energy and Power>Work and Power

A mass of

2 kg

, initially at a height of

1.2 m

above an uncompressed spring with spring constant

2 \times 10^{4} N m^{- 1},

is released from rest to fall on the spring. Taking the acceleration due to gravity as

10 m s^{- 2}

and neglecting the air resistance, the compression of the spring in

mm

MEDIUM

Physics>Mechanics>Work, Energy and Power>Work and Power

The graphs below show the magnitude of the force on a particle as it moves along the positive $X$ -axis from the origin to $X = X_{1}$ . The force is parallel to the $X$ -axis and conservative. The maximum magnitude $F_{1}$ has the same value for all graphs. Rank the situations according to the change in the potential energy associated with the force, least (or most negative) to greatest (or most positive).

Question Image

MEDIUM

Physics>Mechanics>Work, Energy and Power>Work and Power

A particle is released from a height

H

. At a certain height its kinetic energy is half of its potential energy with reference to the surface of the earth. Height and speed of the particle at that instant are respectively

EASY

Physics>Mechanics>Work, Energy and Power>Work and Power

Hydrogen ion and singly ionized helium atom are accelerated, from rest, through the same potential difference. The ratio of final speeds of hydrogen and helium ions is close to:

HARD

Physics>Mechanics>Work, Energy and Power>Work and Power

Consider two masses with

m_{1} > m_{2}

connected by a light inextensible string that passes over a pulley of radius

R

and moment of inertia

I

about its axis of rotation. The string does not slip on the pulley and the pulley turns without friction. The two masses are released from rest separated by a vertical distance

2 h .

When the two masses pass each other, the speed of the masses is proportional to

EASY

Physics>Mechanics>Work, Energy and Power>Work and Power

The potential energy of a system increases if work is done

A particle moves along a curve of unknown shape but magnitude of force F is constant and always acts along tangent to the curve. Then

Important Questions on Work, Energy and Power

Learn and Prepare for any exam you want !