A plane mirror $50 \mathrm{cm}$ long, is hung on a vertical wall of a room, with its lower edge $50 \mathrm{cm}$ above the ground. A man stands in front of the mirror at a distance $2 \mathrm{m}$ away from the mirror. If his eyes are at a height $1.8 \mathrm{m}$ above the ground, then the length (distance between the extreme points of the visible region perpendicular to the mirror) of the floor visible to him due to reflection from the mirror is $\frac{x}{26} \mathrm{m}$. Find the value of $x$.

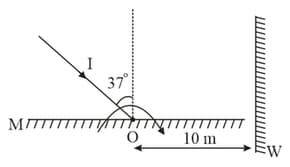
A light ray $I$ is an incident on a plane mirror $M$. The mirror is rotated in the direction as shown in the figure by an arrow at frequency $9/\mathrm{\pi } \mathrm{rps}$. The light reflected by the mirror is received on the wall $W$ at a distance $10 \mathrm{m}$ from the axis of rotation. When the angle of incidence becomes $37°$ the speed of the spot (a point) on the wall is $v\times {10}^2 \mathrm{m} {\mathrm{s}}^{-1}$. Find the value of $v$.

A convex mirror and a concave mirror each of focal length $10 \mathrm{cm}$ are placed coaxially. They are separated by $40 \mathrm{cm}$ and their reflecting surfaces face each other. A point object is kept on the principal axis at a distance $x \mathrm{cm}$ from the concave mirror such that the final image after two reflections, first on the concave mirror, is on the object itself. Write the value of $x$ to the nearest integer.

A point object is placed on the principal axis of a concave mirror of radius of curvature $20 \mathrm{cm}$ at a distance $31 \mathrm{cm}$ from the pole of the mirror. A glass slab of thickness $3 \mathrm{cm}$ and refractive index $1.5$ is placed between object and mirror as shown in the figure.

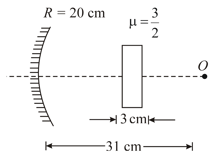
Find the distance $\left(\text{in} \mathrm{cm}\right)$ of the final image formed by the system from the mirror.

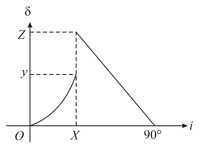
Light is incident from glass to air. The variation of the angle of deviation $\delta$ with the angle of incidence $i$ for $0<i<90°$ is shown. The refractive index of glass is $\frac{2}{\sqrt{3}}$. If the value of $\left(x+y+z\right)$ is $\frac{n\mathrm{\pi }}{6}$, then find the value of $n$.

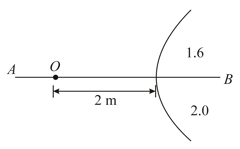
In the figure shown a point object $O$ is placed in the air. The spherical boundary of the radius of curvature $1.0 \mathrm{m}$ separates two media. $AB$ is the principal axis. The refractive index above $AB$ is $1.6$ and below $AB$ is $2.0$. Find the separation between the images $\left(\mathrm{in} \mathrm{m}\right)$ formed due to refraction at the spherical surface.

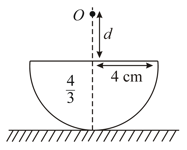
A glass hemisphere of refractive index $\frac{4}{3}$ and of the radius $4 \mathrm{cm}$ is placed on a plane mirror. A point object is placed on the axis of this sphere at a distance $d$ from $O$ as shown in the figure. If the final image is formed at infinity, then find the value of $d \mathrm{in} \mathrm{mm}$

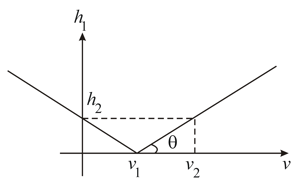
An object of height $h_0=1 \mathrm{cm}$ is moved along the principal axis of a convex lens of focal length $f=10 \mathrm{cm}$. The figure shows the variation of the magnitude of the height of the image with image distance $\left(v\right)$. Find $\left(v_2–v_1\right) \mathrm{in} \mathrm{cm}$.

In the given figure an object $O$ is kept in the air in front of a thin plano-convex lens of the radius of curvature $10 \mathrm{cm}$. Its refractive index is $\frac{3}{2}$ and the medium towards the right of the plane surface is the water of refractive index $\frac{4}{3}$. What should be the distance $x \left(\mathrm{in} \mathrm{cm}\right)$ of the object so that the rays become parallel finally.