If ∮ S E → · d S → = 0 over a surface, then:

The number of flux lines entering the surface must be equal to the number of flux lines leaving it.

The electric field inside the surface is necessarily uniform.

The magnitude of electric field on the surface is constant.

All the charges must necessarily be inside the surface.

EASY

Earn 100

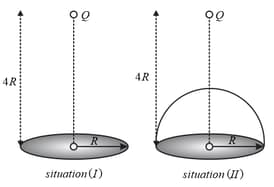
A positive point charge $Q$ is placed (on the axis of the disc) at a distance of $4 R$ above the center of a disc of radius $R$ as shown in situation $I .$ The magnitude of electric flux through the disc is $ϕ .$ Now, a hemispherical shell of a radius $R$ is placed over the disc such that it forms a closed surface as shown in situation $II .$ The flux through the curved surface in the situation $II$ taking direction of area vector along outward normal as positive is

50% studentsanswered this correctly

Important Questions on Electrostatics

EASY

Physics>Electricity and Magnetism>Electrostatics>Gauss’s Law

A charge

q

is enclosed by a Gaussian spherical surface of radius

R

. If the radius is doubled, then the outward electric flux will

EASY

Physics>Electricity and Magnetism>Electrostatics>Gauss’s Law

Consider the charged cylindrical capacitor. The magnitude of electric field

\vec{E}

in its annular region

EASY

Physics>Electricity and Magnetism>Electrostatics>Gauss’s Law

Choose the correct alternative $(1)$ , $(2)$ , $(3)$ or $(4)$ for each of the questions given below $:$

A closed surface in vacuum encloses charges $- q$ and $+ 3 q$ . The total electric flux emerging out of the surface is $:$

EASY

Physics>Electricity and Magnetism>Electrostatics>Gauss’s Law

A charge

+ q

is at a distance

\frac{L}{2}

above a square of side

L

. Then what is the flux linked with the surface?

EASY

Physics>Electricity and Magnetism>Electrostatics>Gauss’s Law

A point charge $q$ is placed at the corner of a cube of side $a$ as shown in the figure. What is the electric flux through the face $A B C D$ ?

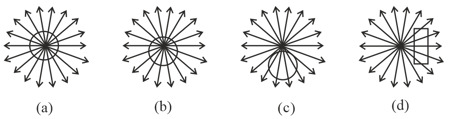
Question Image

EASY

Physics>Electricity and Magnetism>Electrostatics>Gauss’s Law

A hollow insulated conducting sphere is given a positive charge of

10 μC

. What will be the electric field at the centre of the sphere? The radius of the sphere is

2 m

EASY

Physics>Electricity and Magnetism>Electrostatics>Gauss’s Law

The black shapes in the figure below are closed surfaces. The electric field lines are in red. For which case, the net flux through the surfaces is non-zero?

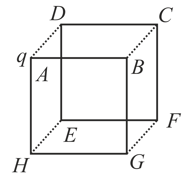
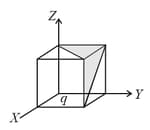
Question Image

EASY

Physics>Electricity and Magnetism>Electrostatics>Gauss’s Law

A charged particle moves with a velocity

v

in a circular path of radius

R

around a long uniformly charged conductor, then

EASY

Physics>Electricity and Magnetism>Electrostatics>Gauss’s Law

A charge $q$ is placed at one corner of a cube as shown in figure. The flux of electrostatic field $\vec{E}$ through the shaded area is:

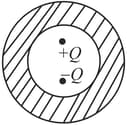
Question Image

EASY

Physics>Electricity and Magnetism>Electrostatics>Gauss’s Law

A point charge of

3.0 μ C

is at the center of a Gaussian surface of radius

10 cm .

What is the net electric flux through the surface?
[use

ε_{0} = 9 \times 10^{- 12}

S . I .

unit]

EASY

Physics>Electricity and Magnetism>Electrostatics>Gauss’s Law

A charge

Q

is placed at a distance

\frac{a}{2}

above the centre of a square surface of side length

a

. The electric flux through the square surface due to the charge would be?
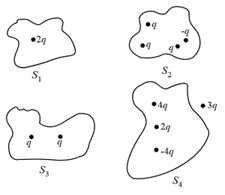
Question Image

EASY

Physics>Electricity and Magnetism>Electrostatics>Gauss’s Law

The magnitude of the average electric field normally present in the atmosphere just above the surface of the Earth is about

150 N / C

, directed inward towards the center of the Earth. This gives the total net surface charge carried by the Earth to be : [Given :

\in_{O} = 8.85 \times 1 0^{- 12} C^{2} / {N-m}^{2}, R_{E} = 6.37 \times 1 0^{6} m

]

EASY

Physics>Electricity and Magnetism>Electrostatics>Gauss’s Law

Shown in the figure are two point charges

+ Q

and

- Q

inside the cavity of a spherical shell. The charges are kept near the surface of the cavity on opposite sides of the centre of the shell. If

σ_{1}

is the surface charge on the inner surface and

Q_{1}

net charge on it and

σ_{2}

the surface charge on the outer surface and

Q_{2}

net charge on it then:

Question Image

EASY

Physics>Electricity and Magnetism>Electrostatics>Gauss’s Law

\oint_{S} \vec{E} \cdot d \vec{S} = 0

over a surface, then:

EASY

Physics>Electricity and Magnetism>Electrostatics>Gauss’s Law

If some charge is given to a solid metallic sphere, the field inside remains zero and by Gauss's law all the charge resides on the surface. Suppose now that Colomb's force between two charges varies as

\frac{1}{r^{3}} .

Then, for a charged solid metallic sphere

HARD

Physics>Electricity and Magnetism>Electrostatics>Gauss’s Law

The potential (in volts) of a charge distribution is given by

V (z) = 30 - 5 z^{2}

for

|z| \leq 1 m

V (z) = 35 - 10 |z|

for

|z| \geq 1 m

V (z)

does not depend on x and y. If this potential is generated by a constant charge per unit volume

ρ_{0}

(in units of

ϵ_{0}

) which is spread over a certain region, then choose the correct statement.

HARD

Physics>Electricity and Magnetism>Electrostatics>Gauss’s Law

The region between two concentric spheres of radii 'a' and 'b', respectively (see figure), has volume charge density

ρ = \frac{A}{r}

, where A is a constant and r is the distance from the centre. At the centre of the spheres is a point charge Q. The value of A such that the electric field in the region between the spheres will be constant, is:

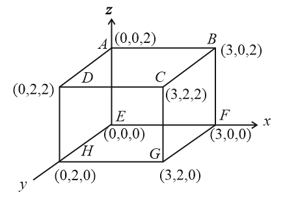
Question Image

EASY

Physics>Electricity and Magnetism>Electrostatics>Gauss’s Law

The electric field in a region is given as,

\vec{E} = (10 \hat{i} + 20 \hat{j}) V m^{- 1}

The net flux passing through a square area of side

2 m

, parallel to

x - z

, plane is,

EASY

Physics>Electricity and Magnetism>Electrostatics>Gauss’s Law

The electric field in a region of space is given by,

\vec{E} = E_{0} \hat{i} + 2 E_{0} \hat{j}

where

E_{0} = 100 N C^{- 1}

. The flux of this field through a circular surface of radius

0.02 m

parallel to the

Y ‐ Z

plane is nearly

EASY

Physics>Electricity and Magnetism>Electrostatics>Gauss’s Law

Four closed surfaces and corresponding charge distributions are shown below.

Question Image

Let the respective electric fluxes through the surfaces be

ϕ_{1}, ϕ_{2}, ϕ_{3}

and

ϕ_{4}

. Then:

Important Questions on Electrostatics

Learn and Prepare for any exam you want !