A rigid body undergoing uniform pure rolling encounters horizontal tracks $AB$ and $BC$ as shown $AB$ is a smooth layer of ice and $BC$ is a rough surface with $\mu =1.$ Both $AB$ and $BC$ are rigid tracks. Which of the following statements are correct?

  

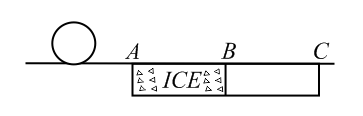
$\left(1\right)$ The body will slow down over $BC$.

$\left(2\right)$ The body will start slipping on $AB$.

$\left(3\right)$ The body remains in pure rolling over the whole stretch $AC$.

$\left(4\right)$ The angular velocity of the body remains constant over the whole stretch $AC$.

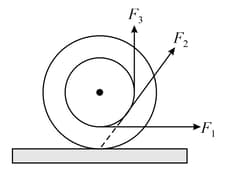
A spool is resting on a horizontal rough table. Forces $F_1, F_2$ and $F_3$ are applied separately as shown. $F_1, F_2, F_3$ all are applied tangentially to the inner circle. $F_1$ is horizontal, $F_3$ is vertical and $F_2$ is passing through the point of contact of spool and table. The correct statement is (assuming that there is no sliding)?

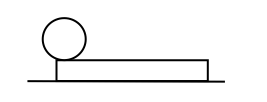
A cylinder of mass $m$ is placed on the edge of a plank of the same mass and length $4\mu g^2$ placed on the smooth horizontal surface, where $\mu$is the coefficient of friction between cylinder and plank and $t$ is the time at which pure rolling starts. The cylinder is given an impulse at, $t=0$ which imparts it a velocity $V_0(\mathrm{m} {\mathrm{s}}^{-1})$. The time in which pure rolling starts will be

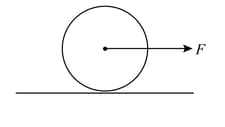
A uniform disc of radius $R$ is resting on a table on its rim. The coefficient of friction between disc and table is $\mu$ (see figure). Now, the disc is pulled with a force $F$ as shown in the figure. What is the maximum value of $F$ for which the disc rolls without slipping?

A $2\mathrm{ }\mathrm{kg}$ uniform cylinder is placed on a plank of mass $4\mathrm{ }\mathrm{kg}$ which in turn rests on a smooth horizontal plane. A constant horizontal force of $20\mathrm{ }\mathrm{N}$ is applied on the plank. If no slipping occurs between cylinder and plank obtain the acceleration of the cylinder and the plank.

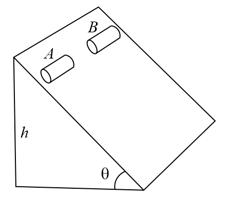
Two cylinders $A$ and $B$ are released on a fixed rough inclined plane of angle $\theta$ with the horizontal from height $‘h’$. $A$ is a hollow cylinder and $B$ is a solid cylinder. Both perform pure rolling. Find the distance between the two cylinders when one of them reaches the bottom. Assume that the axis of both cylinders is parallel to the edge $CD$ always

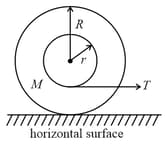
A spool of mass $M=3 \mathrm{kg}$ and radius $R = 20 \mathrm{cm}$ has an axle of radius $r=10 \mathrm{cm}$ around which a string is wrapped. The moment of inertia about an axis perpendicular to the plane of the spool and passing through the center is $\frac{M{R}^2}{2}$. Coefficient of friction between the surface and the spool is $0.4$. Find maximum value of the tension $T$ (in $\mathrm{N}$) that can be applied so that the spool rolls without slipping. $\left(g=10 \mathrm{m} {\mathrm{s}}^{-2}\right)$

A wheel starts rolling with an angular velocity and initial linear velocity up an inclined rough plane of an angle $\theta$ with horizontal and coefficient of friction $\mu$. The linear deceleration is given by:

A wheel of radius $r$ starts rolling with an angular velocity $\omega$ and initial linear velocity $V_0$ up and inclined smooth plane. The wheel will stop going up in time: