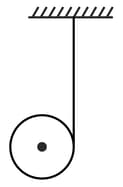
A solid cylinder of mass $3\mathrm{kg}$ and radius $R$ is arranged as shown in figure. A string is wrapped over the cylinder. The system is released from rest. The tension in the string is ______ newton. $\left(g=10 \mathrm{m} {\mathrm{s}}^{-2}\right)$

 

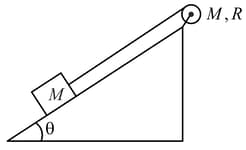
A block of mass $M$ is released from rest on top of a smooth inclined plane of inclination $\theta$. The block is connected to a light string which is wound over a disc shaped pulley of mass $M$ and radius $R$ as shown. If length of inclined surface is $l$, then time (in second) taken by the block to reach the bottom (Given $\frac{l}{g\sin \theta }=3$, in S.I. unit)

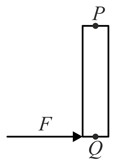
A force $F$ acts at the end $Q$ of a uniform rod placed at rest on a smooth horizontal plane. If ratio of magnitude of initial acceleration of the point $P$ and $Q$, is $1:k$, then value of $k$ is ______.

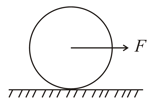
A uniform solid sphere of mass $M$ is at rest on a rough horizontal surface. The coefficient of friction between ground and sphere is $\mu$. Force $F$ is applied horizontally such that its line of action passes through the centre of the sphere. If maximum value of $F$, so that the sphere will not slip, is equal to $\frac{N}{4}\mu \mathrm{Mg}$, then value of $N$ is _____.

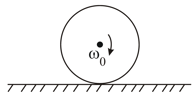
A disc of radius $R$ rotating with an angular velocity ${\omega }_0$ is placed on a rough horizontal surface. If initial velocity of centre of disc is zero, then velocity of centre of disc when it ceases to slip, comes out to be $\frac{{\omega }_{0}R}{k}$, value of $k$ is _____.

Two men of masses $60 \mathrm{kg}$ and $80 \mathrm{kg}$ are at the two ends of a slab of mass $160 \mathrm{kg}$ as shown in figure. The horizontal surface is smooth. If the men interchange their positions on the slab, then displacement (in $\mathrm{cm}$) of the slab is approximately (closest integer)

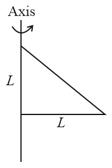
The moment of inertia of a uniform triangular plate of mass $M$ about the axis shown in the figure is $\frac{M{L}^2}{k}$, value of $k$ is _____.

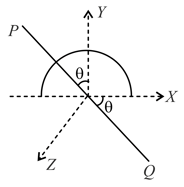
A uniform semicircular disc of mass $2\mathrm{kg}$ and radius $R=2\mathrm{m}$ lies in $x-y$ plane with its centre at origin as shown in figure. Also, axis $PQ$ is in the $x-y$ plane. Then, the moment of inertia (in S.I. unit) of semicircular disc about axis $PQ$ is

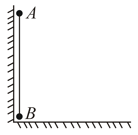
Two identical small balls $A$ and $B$ are connected at ends of a light rod and placed vertically as shown in figure. Friction is absent everywhere. By small disturbance, the ball $B$ slides away from the wall. Find angle made by the rod with the horizontal, at the moment ball $A$ breaks off from the vertical wall