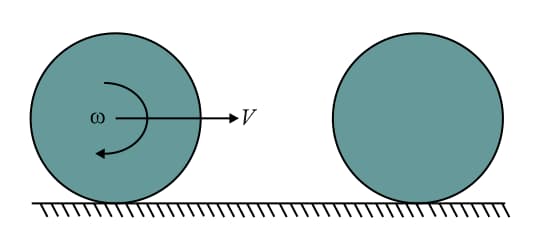
A solid sphere is rolling without slipping on a rough ground as shown in figure. It collides elastically with an identical another sphere at rest. There is no friction between the two spheres. Radius of each sphere is $R$ and mass is $m$. The linear velocity of first sphere after it again starts rolling without slipping is,

 

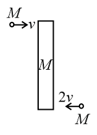
Two masses $\left(M\right)$ are moving perpendicular to the rod of mass $M$ with velocities $v\text{ and} 2v$ as shown in the figure. The length of the rod is $L$. Find the ratio of the final velocity of the rod and its angular velocity if the masses stop at their place after collision.

A uniform smooth rod (mass $m$ and length $\mathcal{l}$ ) placed on a smooth horizontal floor is hit by a particle (mass $m$) moving on the floor, at a distance $\frac{\mathcal{l}}{4}$ from one end elastically ($e=1$). The distance travelled by the centre of the rod after the collision, when it has completed three revolutions, will be

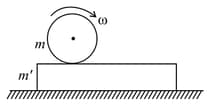
A uniform solid cylinder is given an angular speed $\omega$ and placed on a rough plate of negligible thickness. The horizontal surface below the plate is smooth. Then the angular speed of the cylinder when it starts pure rolling on the plate will be: [Assume sufficient length of plate]

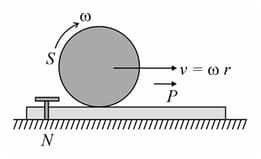
A sphere $S$ rolls without slipping, moving with a constant speed on a plank $P$. The friction between the upper surface of $P$ and the sphere is sufficient to prevent slipping, while the lower surface of $P$ is smooth and rests on the ground. Initially, $P$ is fixed to the ground by a pin $N$. If $N$ is suddenly removed, 

For next 2 question please follow the same

A disc of mass m and radius R is placed over a plank of same mass m. There is sufficient friction between disc and plank to prevent slipping. A force F is applied at the centre of the disc.

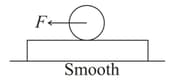
Acceleration of the plank is