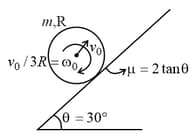
A solid sphere of mass $m=3 \mathrm{kg}$ and radius $R=10 \mathrm{cm}$ is projected up an inclined plane of inclination $\theta =30°$ with an initial velocity $V_0=15 {\mathrm{ms}}^{-1}$ and initial angular velocity ${\omega }_0=\frac{V_0}{3R}$ as shown. The coefficient of friction between the sphere and inclined plane is $\mu =2\tan \theta$. Then find the velocity (in $\mathrm{m} {\mathrm{s}}^{-1}$ ) of the sphere, when it will rolling without slipping relative to the inclined plane.

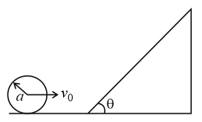
A sphere of radius $a$ and mass $m$ rolls along a horizontal plane with constant speed $v_0$. It encounters an inclined plane at angle $\theta$ and climbs upward. Assuming that it rolls without slipping, how far up the sphere will travel?

A small roller of diameter $20 \mathrm{cm}$ has an axle of diameter $10 \mathrm{cm}$ (see figure below on the left). It is on a horizontal floor and a meter scale is positioned horizontally on its axle with one edge of the scale on top of the axle (see figure on the right). The scale is now pushed slowly on the axle so that it moves without slipping on the axle, and the roller starts rolling without slipping. After the roller has moved $50 \mathrm{cm},$ the position of the scale will look like (figures are schematic and not drawn to scale)

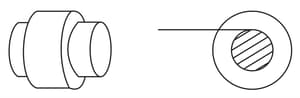
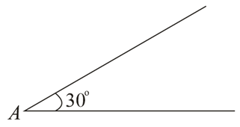
A sphere of mass $2 \mathrm{kg}$ and radius $0.5 \mathrm{m}$ is rolling with an initial speed of $1{ \mathrm{m} \mathrm{s}}^{-1}$ goes up an inclined plane which makes an angle of $30°$ with the horizontal plane, without slipping. How low will the sphere take to return to the starting point $A$?

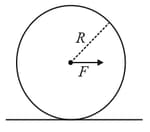
A horizontal force $F$ is applied at the centre of mass of a cylindrical object of mass $m$ and radius $R$, perpendicular to its axis as shown in the figure. The coefficient of friction between the object and the ground is $\mu$. The centre of mass of the object has an acceleration $a$. The acceleration due to gravity is $g$. Given that the object rolls without slipping, which of the following statement(s) is (are) correct?