A uniform disc of radius $R$ is spinned to the angular velocity $\omega$ and then carefully placed on a horizontal surface. How long will the disc be rotating on the surface, if the friction coefficient is equal to $k$? The pressure exerted by the disc on the surface can be regarded as uniform.

A uniform cylinder, of radius $R$ and mass $M$, can rotate freely about a stationary horizontal axis $O$. A thin cord, of length $l$ and mass $m$, is wound on the cylinder in a single layer. Find the angular acceleration of the cylinder as a function of the length $x$ of the hanging part of the cord. The wound part of the cord is supposed to have its centre of gravity on the cylinder axis.

A uniform cylinder of mass $m=8.0 \mathrm{kg}$, and radius $R=1.3 \mathrm{cm}$, starts descending, at a moment $t=0$, due to gravity. Neglecting the mass of the thread, find:

$\left(\mathrm{a}\right)$ the tension of each thread and the angular acceleration of the cylinder;
$\left(\mathrm{b}\right)$ the time dependence of the instantaneous power developed by the gravitational force.

$\left(\text{Gravitational acceleration} g=9.8 \mathrm{m} {\mathrm{s}}^{-2}\right)$

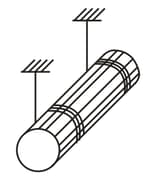
Thin threads are tightly wound on the ends of a uniform solid cylinder, of mass $m$. The free ends of the threads are attached to the ceiling of an elevator car. The car starts going up with an acceleration ${\omega }_0$. Find the acceleration $\omega \text{'}$, of the cylinder, relative to the car, and the force $F$, exerted by the cylinder on the ceiling (through the threads).

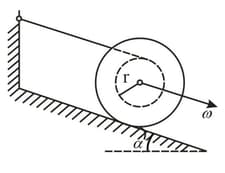
A spool, with a thread wound on it, is placed on an inclined smooth plane, set at an angle $\alpha =30°$, to the horizontal. The free end of the thread is attached to the wall, as shown in the figure. The mass of the spool is $m=200 \mathrm{g}$, its moment of inertia, relative to its own axis, is $I=0.45 \mathrm{g} {\mathrm{m}}^2$ and the radius of the wound thread layer is $r=3.0 \mathrm{cm}$. Find the acceleration of the spool axis.

$\left(\text{Gravitational acceleration }g=9.8 \mathrm{m} {\mathrm{s}}^{-2}\right)$

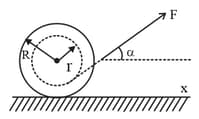
A spool, with a thread wound on it, of mass $m$, rests on a rough horizontal surface. Its moment of inertia, relative to its own axis, is equal to, $I=\gamma m{R}^2$, where $\gamma$ is a numerical factor and $R$ is the outside radius of the spool. The radius of the wound thread layer is equal to $r$. The spool is pulled, without sliding, by the thread, with a constant force $F$, directed at an angle $\alpha$ to the horizontal. Find:
$\left(a\right)$ the projection of the acceleration vector of the spool axis, on the $x$-axis;
$\left(b\right)$ the work performed by the force $F$, during the first $t \mathrm{second}$, after the beginning of the motion.

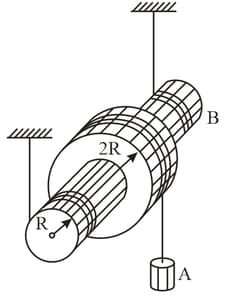
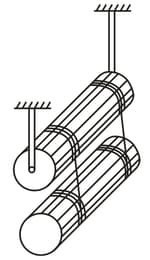
The arrangement shown in figure consists of two identical uniform solid cylinders, each of mass $m$, on which two light threads are wound symmetrically. Find the tension of each thread in the process of motion. The friction in the axle of the upper cylinder is assumed to be absent.

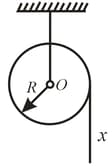
In the arrangement shown in the figure, a weight $A$, possesses mass $m$. A pulley $B$, possesses mass $M$. Also known are the moment of inertia $I$, of the pulley relative to its axis and the radii of the pulley $R$ and $2R$. The mass of the threads is negligible. Find the acceleration of the weight $A$, after the system is set free.