MEDIUM

Earn 100

At what height from the surface of earth, the value of 'g' is the same as that at a depth of $160 km$ below the surface of the earth? The radius of the earth is $6400 km$ .

Important Questions on Gravitation

EASY

Physics>Mechanics>Gravitation>Simple Pendulum

Starting from the center of the earth having radius,

R

, the variation of

g

(acceleration due to gravity) is shown by

MEDIUM

Physics>Mechanics>Gravitation>Simple Pendulum

Kepler's third law states that the square of the period of revolution

(T)

of a planet around the sun is proportional to the third power of the average distance

r

between sun and planet i.e.,

T^{2} = K r^{3}

, here

K

is constant. If the masses of sun and planet are

M

and

m

respectively then as per Newton's law of gravitation force of attraction between them is

F = \frac{G M m}{r^{2}}

, here

G

is gravitational constant. The relation between

G

and

K

is described as:

EASY

Physics>Mechanics>Gravitation>Simple Pendulum

Suppose that the angular velocity of rotation of the Earth is increased. Then, as a consequence,

MEDIUM

Physics>Mechanics>Gravitation>Simple Pendulum

The depth

d

at which the value of acceleration due to gravity becomes

\frac{1}{n}

times the value at the earth's surface is

(R

= radius of the earth

)

HARD

Physics>Mechanics>Gravitation>Simple Pendulum

A very long (length $L$ ) cylindrical galaxy is made of uniformly distributed mass and has radius $R (R < < L)$ . A star outside the galaxy is orbiting the galaxy in a plane perpendicular to the galaxy and passing through its centre. If the time period of the star is $T$ and its distance from the galaxy's axis is $r$ , then

EASY

Physics>Mechanics>Gravitation>Simple Pendulum

The variation of acceleration due to gravity

g

with distance

d

from the centre of the earth is best represented by (

R

= Earth's radius):

MEDIUM

Physics>Mechanics>Gravitation>Simple Pendulum

Two particles of the same mass

m

are moving in circular orbits because of force, given by

F (r) = - \frac{16}{r} - r^{3}

. The first particle is at a distance

r = 1

, and the second, at

r = 4

. The best estimate for the ratio of kinetic energies of the first and the second particle is closest to

MEDIUM

Physics>Mechanics>Gravitation>Simple Pendulum

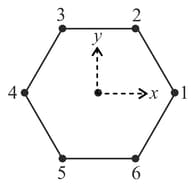
Six objects are placed at the vertices of $a$ regular hexagon. The geometric centre of the hexagon is at the origin with objects $1$ and $4$ on the $X$ -axis (see figure). The mass of the $k$ th object is $m_{k} = k^{i} M |{cosθ}_{k}|,$ where $i$ is an integer, $M$ is $a$ constant with dimension of mass and $θ_{k}$ is the angular position of the $k$ th vertex measured from the positive $x$ -axis in the counter-clockwise sense. If the net gravitational force on $a$ body at the centroid vanishes, the value of $i$ is

Question Image

MEDIUM

Physics>Mechanics>Gravitation>Simple Pendulum

Four particles, each of mass

M

and equidistant from each other, move along a circle of radius

R

under the action of their mutual gravitational attraction. The speed of each particle is

HARD

Physics>Mechanics>Gravitation>Simple Pendulum

Two satellites

S_{1}

and

S_{2}

are revolving around a planet in the opposite sense in coplanar circular concentric orbits. At time

t = 0,

the satellites are farthest apart. The periods of revolution of

S_{1}

and

S_{2}

are

3 h

and

24 h

respectively. The radius of the orbit of

S_{1}

3 \times 10^{4} km .

Then the orbital speed of

S_{2}

as observed from

MEDIUM

Physics>Mechanics>Gravitation>Simple Pendulum

A ball is launched from the top of Mount Everest which is at an elevation of

9000 m

. The ball moves in a circular orbit around the earth. The acceleration due to gravity near the earth's surface is

g

. The magnitude of the ball's acceleration while in orbit is,

HARD

Physics>Mechanics>Gravitation>Simple Pendulum

A straight rod of length

L

extends from

x = a

x = L + a .

The gravitational force it exerts on a point mass 'm' at

x = 0,

if the mass per unit length of the rod is

A + B x^{2},

is given by:

MEDIUM

Physics>Mechanics>Gravitation>Simple Pendulum

The International space station is maintained in a nearly circular orbit with a mean altitude of

330 km

and a maximum of

410 km

. An astronaut is floating in the space station's cabin. The acceleration of astronauts as measured from the earth is-

EASY

Physics>Mechanics>Gravitation>Simple Pendulum

If radius of the Earth contracts by

2 %

and its mass remains the same, then the weight of a body at the Earth's surface

EASY

Physics>Mechanics>Gravitation>Simple Pendulum

Two planets

A

and

B

have the same average density. Their radii

R_{A}

and

R_{B}

are such that

R_{A} : R_{B} = 3 : 1

. If

g_{A}

and

g_{B}

are the acceleration due to gravity at the surfaces of the planets, the

g_{A} : g_{B}

equals

HARD

Physics>Mechanics>Gravitation>Simple Pendulum

The mass density of a spherical body is given by

ρ (r) = \frac{k}{r}

for

r \leq R

and

ρ (r) = 0

for

r > R,

where

r

is the distance from the center. The correct graph that describes qualitatively the acceleration,

a

of a test particle as a function of

r

is:

MEDIUM

Physics>Mechanics>Gravitation>Simple Pendulum

If the Earth has no rotational motion, the weight of a person on the equator is

W

. Determine the speed with which the earth would have to rotate about its axis so that the person at the equator will weigh

\frac{3}{4} W

. The radius of the Earth is

6400 km

and

g = 10 m s^{- 2}

EASY

Physics>Mechanics>Gravitation>Simple Pendulum

A star mass $M$ (equal to the solar mass) with a planet (much smaller than the star) revolves around the star in a circular orbit. The velocity of the star with respect to the centre of mass of star-planet system is shown below:

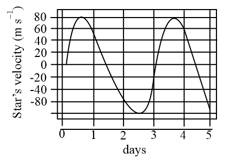
Question Image

The radius of the planet's orbit is closest to ( $1 AU = Distance Earth and Sun$ )

EASY

Physics>Mechanics>Gravitation>Simple Pendulum

If the change in the value of

g

at a height

h

above the surface of the earth is the same as at a depth

x

below it, then (both

x

and

h

being much smaller than the radius of the earth)

EASY

Physics>Mechanics>Gravitation>Simple Pendulum

At what height from the surface of earth the gravitation potential and the value of g are

- 5.4 \times 10^{7} J k g^{- 2}

and

6.0 m s^{- 2}

respectively? Take the radius of earth as

6400 km

At what height from the surface of earth, the value of 'g' is the same as that at a depth of 160 km below the surface of the earth? The radius of the earth is 6400 km.

Important Questions on Gravitation

Learn and Prepare for any exam you want !

At what height from the surface of earth, the value of 'g' is the same as that at a depth of $160 km$ below the surface of the earth? The radius of the earth is $6400 km$ .