Define constructive interference.
Important Questions on Waves and Sound
Out of the given four waves and
emitted by four different sources and respectively, interference phenomena would be observed in space under appropriate conditions when
Two pulses travel in mutually opposite directions in a string with a speed of as shown in the figure. Initially the pulses are apart. What will be the state of the string after two seconds?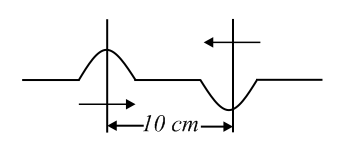
Two waves of same frequency have amplitudes . These waves are made to superpose in the same direction. The ratio of maximum intensity to minimum intensity at various places will be
Four different independent waves are represented by
(i)
(ii)
(iii)
(iv)
With which two waves, interference is possible?
When interference is produced by two progressive waves of equal frequencies, then the maximum intensity of the resulting sound are times the intensity of each of the component waves. The value of is,
And
Here x and y are in m and t is in s.
The number of maximum heard in one second will be
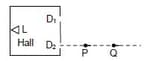
A loudspeaker is placed in the hall with two doors and as shown below. The distance between and is . The loudspeaker is equidistant from and Monotonic sound waves are emitted from the loudspeaker, and it is found that at point which is from and at point the sound intensities are minimum. The line joining and is perpendicular to the line joining and . No other minimum intensity locations can be found between and beyond along the line. What is the frequency of the sound wave generated by the loudspeaker ?(Speed of sound is )
Two waves having equations . If in the resultant wave the frequency and amplitude remain equal to those of superimposing waves. Then phase difference between them is