Find the relation between the magnetic field at on axis, and magnetic field at the center of coil. Here is radius of the coil.

Important Questions on Moving Charges and Magnetism
A compass needle free to turn in a horizontal plane is placed at the centre of circular coil of turns and radius . The coil is in a vertical plane making an angle of with the magnetic meridian. When the current in the coil is , the needle points west to east. Determine the horizontal component of the Earth's magnetic field at the location.
Next, the current in the coil is reversed and the coil is rotated about its vertical axis by an angle of in the anti clockwise sense looking from above. Predict the direction of the needle. Take the magnetic declination at the places to be zero.
Show that this reduces to the familiar result for the field at the centre of the coil.
The figure shows two wires, each carrying a current. The wire consists of a circular arc of radius and two radial lengths; it carries current in the direction indicated. The wire is long and straight; it carries a current that can be varied, and it is at a distance of from the Centre of the arc. The net magnetic field due to the two currents is measured at the Centre of curvature of the arc. Figure is a plot of the component of in the direction perpendicular to the figure as a function of current . What is the angle subtended by the arc?
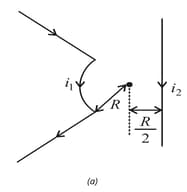
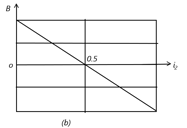
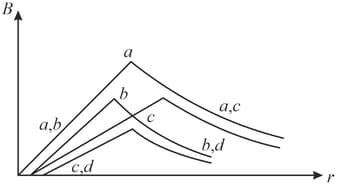
Which wire has the largest radius?
