MEDIUM
JEE Main/Advance
IMPORTANT
Earn 100
In the arrangement shown, what is the expression for emf induced in the smaller loop of radius The current varies as
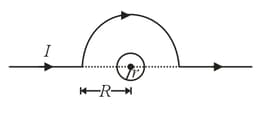

(a)
(b)
(c)
(d)Zero
33.33% studentsanswered this correctly

Important Questions on Electromagnetic Induction
HARD
JEE Main/Advance
IMPORTANT
A uniformly wound solenoid coil of self-inductance and resistance is broken up into two identical coils. These identical coils are then connected in parallel across a battery of negligible resistance. The time constant for the current in the circuit is
EASY
JEE Main/Advance
IMPORTANT
An inductor of inductance is decayed through a resistance A radioactive sample decays with an average life The value of for which the electric energy stored in the inductor to the activity of radioactive sample remains constant
EASY
JEE Main/Advance
IMPORTANT
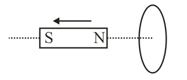
A bar magnet with its north (N) and south (S) poles is initially moving to the left, along the axis of fixed circular conducting loop as shown. A current is induced in the loop. As seen from the magnet side
MEDIUM
JEE Main/Advance
IMPORTANT
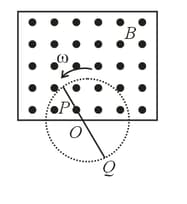
A uniform and constant magnetic field coming out of the plane of the paper exists in a rectangular region as shown in figure. conducting rod is rotated about its mid-point with a uniform angular speed in the plane of the paper. The emf induced between and is best represented by the graph
HARD
JEE Main/Advance
IMPORTANT
According to Faraday's law of electromagnetic induction, the total charge flown through a crosssection of a conducting loop depends upon
HARD
JEE Main/Advance
IMPORTANT
The mutual induction between primary and secondary coils of a transformer is . If an current of peak value and frequency flows through the primary coil, then the peak voltage in the secondary coil is,
MEDIUM
JEE Main/Advance
IMPORTANT
In the given circuit, the switch is closed at The ratio of electric current through resistance at and at is
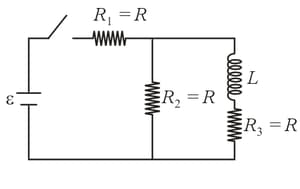
EASY
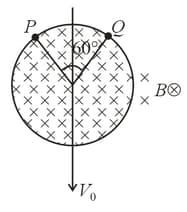
JEE Main/Advance
IMPORTANT
A conducting ring of radius a falls vertically downward with a velocity in a horizontal magnetic field The potential difference between two points and located symmetrically on both sides of the vertical will be