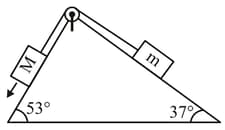
In the given arrangement of a doubly inclined plane two blocks of masses $M$ and $m$ are placed. The blocks are connected by a light string passing over an ideal pulley as shown. The coefficient of friction between the surface of the plane and the blocks is $0.25$. The value of $m$, for which $M=10 \mathrm{kg}$ will move down with an acceleration of $2 \mathrm{m} {\mathrm{s}}^{-2}$, is : (take $g=10 \mathrm{m} {\mathrm{s}}^{-2}$ and $\tan 37°=\frac{3}{4}$)

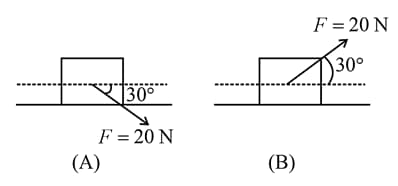
A block of mass $5 \mathrm{k}\mathrm{g}$ is (i) pushed in case $\left(\mathrm{A}\right)$ and (ii) pulled in case $\left(\mathrm{B}\right),$ by a force $\mathrm{F}=20\mathrm{ }\mathrm{N},$ making an angle of ${30}^o$ with the horizontal, as shown in the figures. The coefficient of friction between the block and floor is $\mu =0.2.$ The difference between the accelerations of the block, in case $\left(\mathrm{B}\right)$ and case $\left(\mathrm{A}\right)$ will be:
$\left(\mathrm{g}=10\mathrm{m}\mathrm{ }{\mathrm{s}}^{-2}\right)$

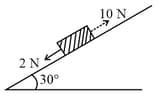
A block of mass $5 \mathrm{kg}$ resting on a horizontal surface is connected by a cord, passing over a light frictionless pulley to a hanging block of mass $5 \mathrm{kg}$. The coefficient of kinetic friction between the block and the surface is $0.5$. Tension in the cord is $\left(g=9.8 \mathrm{m} {\mathrm{s}}^{-2}\right)$

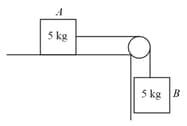
Consider the system shown below.

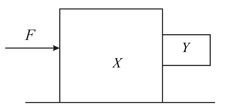
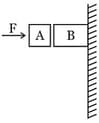
A horizontal force $F$ is applied to a block $X$ of mass $8 \mathrm{kg}$ such that the block $Y$ of mass $2 \mathrm{kg}$ adjacent to it does not slip downwards under gravity. There is no friction between the horizontal plane and the base of the block $X$. The coefficient of friction between the surfaces of the blocks $X$ and $Y$ is $0.5$. Take acceleration due to gravity to be $10 \mathrm{m} {\mathrm{s}}^{-2}$. The minimum value of $F$ is

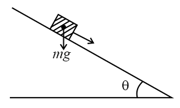
A block of mass $5 \mathrm{kg}$ is kept against an accelerating wedge with a wedge angle of $45°$ to the horizontal. The co-efficient of friction between the block and the wedge is $\mu =0.4$. What is the minimum absolute value of the acceleration of the wedge to keep the block steady. Assume $g=10 \mathrm{m} {\mathrm{s}}^{-2}$