The locus of the point of intersection of the lines 2 x - y + 4 2 k = 0 and 2 k x + k y - 4 2 = 0 ( k is any non-zero real parameter) is

a hyperbola with length of its transverse axis 8 2

an ellipse whose eccentricity is 1 3

a hyperbola whose eccentricity is 3

an ellipse with length of its major axis 8 2

HARD

Earn 100

Let A₀, A₁, A₂, A₃, A₄, A₅ be the vertices of a regular hexagon inscribed in a circle of unit radius. Then, the product of the lengths of the line segments A₀A₁, A₀A₂ , A₀A₄ is

42.86% studentsanswered this correctly

Important Questions on Straight Lines

MEDIUM

Mathematics>Coordinate Geometry>Straight Lines>Basics of 2D Coordinate Geometry

Locus of the image of the point $(2, 3)$ in the line $(2 x - 3 y + 4) + k (x - 2 y + 3) = 0, k \in R,$ is a

MEDIUM

Mathematics>Coordinate Geometry>Straight Lines>Basics of 2D Coordinate Geometry

Let

x_{0}, y_{0}

be fixed real numbers such that

x_{0}^{2} + y_{0}^{2} > 1

. If

x, y

are arbitrary real numbers such that

x^{2} + y^{2} \leq 1,

then the minimum value of

{(x - x_{0})}^{2} + {(y - y_{0})}^{2}

HARD

Mathematics>Coordinate Geometry>Straight Lines>Basics of 2D Coordinate Geometry

In a triangle

A B C

, coordinates of

A

are

(1, 2)

and the equations of the medians through

B

and

C

are respectively,

x + y = 5

and

x = 4

. Then area of

Δ A B C

(in sq. units) is :

HARD

Mathematics>Coordinate Geometry>Straight Lines>Basics of 2D Coordinate Geometry

If a circle of radius

R

passes through the origin

O

and intersects the coordinate axes at

A

and

B

, then the locus of the foot of perpendicular from

O

A B

is :

EASY

Mathematics>Coordinate Geometry>Straight Lines>Basics of 2D Coordinate Geometry

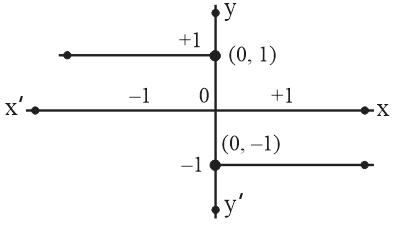
The equation of the graph shown here is:

Question Image

MEDIUM

Mathematics>Coordinate Geometry>Straight Lines>Basics of 2D Coordinate Geometry

Let

S

be the set of all triangles in the

x y

-plane, each having one vertex at the origin and the other two vertices lie on coordinate axes with integral coordinates. If each triangle in

S

has area

50

sq. units, then the number of elements in the set

S

is:

EASY

Mathematics>Coordinate Geometry>Straight Lines>Basics of 2D Coordinate Geometry

P (8, 10)

and

Q (14, - 2)

are two given points and the point

R

divides the line-segment

P Q

externally in the ratio

8 : 6

. The coordinates of

R

are

MEDIUM

Mathematics>Coordinate Geometry>Straight Lines>Basics of 2D Coordinate Geometry

The locus of the point of intersection of the lines

\sqrt{2} x - y + 4 \sqrt{2} k = 0

and

\sqrt{2} k x + k y - 4 \sqrt{2} = 0

(

k

is any non-zero real parameter) is

HARD

Mathematics>Coordinate Geometry>Straight Lines>Basics of 2D Coordinate Geometry

If the distance between the points

(x, 0)

and

(- 7, 0)

10

. Then, the possible values of

x

are ______.

MEDIUM

Mathematics>Coordinate Geometry>Straight Lines>Basics of 2D Coordinate Geometry

P (- 3, - 2, 4), Q (- 9, - 8, 10)

and

R (- 5, - 4, 6)

are collinear, then the ratio in which

R

divides

P Q

HARD

Mathematics>Coordinate Geometry>Straight Lines>Basics of 2D Coordinate Geometry

Let

B C

be a fixed line segment in the plane. The locus of a point

A

such that the triangle

A B C

is isosceles, is (with finitely many possible exceptional points)

MEDIUM

Mathematics>Coordinate Geometry>Straight Lines>Basics of 2D Coordinate Geometry

Find the ratio in which line $3 x + 2 y = 17$ divides the line segment joined by points $(2, 5)$ and $(5, 2) .$

MEDIUM

Mathematics>Coordinate Geometry>Straight Lines>Basics of 2D Coordinate Geometry

Let

A = (a_{1}, a_{2})

and

B = (b_{1}, b_{2})

be two points in the plane with integer coordinates. Which one of the following is not a possible value of the distance between

A

and

B

MEDIUM

Mathematics>Coordinate Geometry>Straight Lines>Basics of 2D Coordinate Geometry

A point

P

moves on the line

2 x - 3 y + 4 = 0 .

Q (1, 4)

and

R (3, - 2)

are fixed points, then the locus of the centroid of

Δ P Q R

is a line:

EASY

Mathematics>Coordinate Geometry>Straight Lines>Basics of 2D Coordinate Geometry

A B

is a straight line and

O

is point on the line

A B

. If one draws a line

O C

not coinciding with

O A

O B

, then the

∠ A O C

and

∠ B O C

are

HARD

Mathematics>Coordinate Geometry>Straight Lines>Basics of 2D Coordinate Geometry

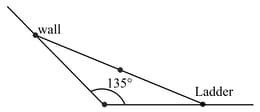
A wall is inclined to the floor at an angle of $135 ° .$ A ladder of length $l$ is resting on the wall. As the ladder slides down, its mid-point traces an arc of an ellipse. Then the area of the ellipse is
Question Image

HARD

Mathematics>Coordinate Geometry>Straight Lines>Basics of 2D Coordinate Geometry

In a circle with centre

O

, suppose

A, P, B

are three points on its circumference such that

P

is the mid-point of minor arc

A B

. Suppose when

∠ A O B = θ, \frac{a r e a (Δ A O B)}{a r e a (Δ A P B)} = \sqrt{5} + 2

∠ A O B

is doubled to

2 θ,

then the ratio

\frac{a r e a (Δ A O B)}{a r e a (Δ A P B)}

is.

EASY

Mathematics>Coordinate Geometry>Straight Lines>Basics of 2D Coordinate Geometry

A straight line through the origin

O

meets the parallel lines

4 x + 2 y = 9

and

2 x + y + 6 = 0

P

and

Q

respectively. The point

O

divides the segment

P Q

in the ratio

HARD

Mathematics>Coordinate Geometry>Straight Lines>Basics of 2D Coordinate Geometry

Find the area of the triangle formed by the lines

x - 3 y = 0, x - y = 4

and

x + y = 4 .

MEDIUM

Mathematics>Coordinate Geometry>Straight Lines>Basics of 2D Coordinate Geometry

Find the area of the triangle formed with the three straight lines represented by:

$(i) x + y = 0 (i i) 3 x = 5 y; a n d (i i i) y = 3 x - 12$

Let A0, A1, A2, A3, A4, A5 be the vertices of a regular hexagon inscribed in a circle of unit radius. Then, the product of the lengths of the line segments A0A1, A0A2 , A0A4 is

Important Questions on Straight Lines

Learn and Prepare for any exam you want !

Let A₀, A₁, A₂, A₃, A₄, A₅ be the vertices of a regular hexagon inscribed in a circle of unit radius. Then, the product of the lengths of the line segments A₀A₁, A₀A₂ , A₀A₄ is