EASY

Earn 100

Match the following ?

Column I Column II

(i) Adiabatic expansion (a) No work done

(ii) Isobaric expansion (b) Constant internal energy

(iii) Isothermal expansion (c) Increase in intemal energy

(iv) Isochoric expansion (d) Decrease in internal energy

50% studentsanswered this correctly

Important Questions on Thermodynamics

EASY

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

Which of the following does not follow first law of thermodynamics?

(w =

work

; q =

heat

; ΔU =

change in internal energy

)

EASY

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

The first law of thermodynamics confirms the law of _____

MEDIUM

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

The $P - V$ diagram shown below indicates two paths along which a sample of gas can be taken from state $A$ to state $B$ . The energy equal to $5 P V$ in the form of heat is required to be transferred if the Path- $1$ is chosen. How much energy in the form of heat should be transferred if Path- $2$ is chosen?

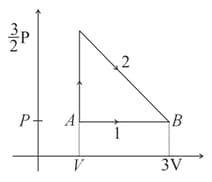
Question Image

MEDIUM

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

A gas undergoes a thermodynamic cycle as shown in pressure-volume diagram below. The heat transferred to the gas is $300 J$ and $140 J$ along path $1 \to 2$ and $2 \to 3$ respectively. The internal energy is changed by $- 260 J$ along path $3 \to 1 .$ The work done by gas along path $1 \to 2 \to 3$ is

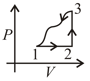
Question Image

EASY

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

A gas can be taken from

A

B

via two different processes

ACB

and

ADB

When path

ACB

is used

60 J

of heat flows into the system and

30 J

of work is done by the system. If the path

ADB

is used then work done by the system is

10 J

, the heat flows into the system in the path

ADB

is:

MEDIUM

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

The internal energy of the air, in a room of volume

V

, at temperature

T

and with outside pressure

P

increasing linearly with time, varies as

MEDIUM

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

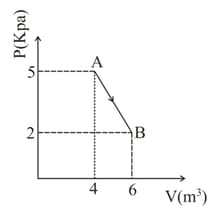
One mole of an ideal diatomic gas undergoes a transition from $A$ to $B$ along a path $A B$ as shown in figure. The change in internal energy of the gas during the transition is

Question Image

MEDIUM

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

The amount of heat needed to raise the temperature of

4 moles

of a rigid diatomic gas from

0 ° C

50 ° C

when no work is done is

(R

is the universal gas constant)

HARD

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

An ideal gas is undergoing a cyclic thermodynamic process in different ways as shown in the corresponding P-V diagrams in column 3 of the table. Consider only the path from state 1 to state 2. W denotes the corresponding work done on the system. The equations and plots in the table have standard notations as used in thermodynamic process. Here

γ

is the ratio of heat capacities at constant pressure and constant volume. The number of moles in the gas is n.

Column – 1	Column – 2	Column – 3
(I) $W_{1 \to 2} = \frac{1}{γ - 1} (P_{2} V_{2} - P_{1} V_{1})$	(i) Isothermal	(P)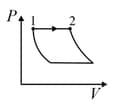
(II) $W_{1 \to 2} = - P V_{2} + P V_{1}$	(ii) Isochoric	(Q)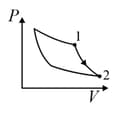
(III) $W_{1 \to 2} = 0$	(iii) Isobaric	(R)
(IV) $W_{1 \to 2} = - n R T \ln (\frac{V_{2}}{V_{1}})$	(iv) Adiabatic	(S)

Which of the following options is the only correct representation of the process

∆ U = ∆ Q - P ∆ V

MEDIUM

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

A sample of an ideal gas is taken through the cyclic process

a b c a

as shown in the figure. The change in the internal energy of the gas along the path

c a

- 180 J .

The gas absorbs

250 J

of heat along the path

a b

and

60 J

along the path

b c

. The work done by the gas along the path

a b c

is:

MEDIUM

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

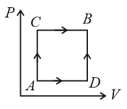
An ideal gas is made to undergo the cyclic process shown in the figure below.
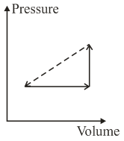
Question Image
Let $Δ W$ depict the work done, $Δ U$ be the change in internal energy of the gas and $Q$ be the heat added to the gas. Sign of each of these three quantities for the whole cycle will be ( $0$ refers to no change)

MEDIUM

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

In a thermodynamic system,

Q

represents the energy transferred to or from a system by heat and

W

represents the energy transferred to or from a system by work

(I)

Q > 0

and

W = 0

(II)

Q < 0

and

W = 0

(III)

W > 0

and

Q = 0

(IV)

W < 0

and

Q = 0

Which of the above will lead to an increase in the internal energy of the system?

EASY

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

In thermodynamics, heat and work are :

HARD

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

Certain quantity of heat is supplied to a monatomic ideal gas which expands at constant pressure. The percentage of heat that is used to do work by the gas is

EASY

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

A given quantity of gas is taken from the state $A$ to the state $C$ reversibly by two paths, $A \to C$ directly and $A \to B \to C$ as shown in the figure below:

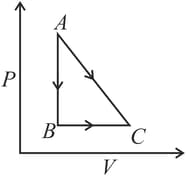
Question Image

During the process $A \to C$ , work done by the gas is $100 J$ and heat absorbed is $120 J$ . If during the process $A \to B \to C$ , the work done by the gas is $80 J$ , the heat absorbed is

EASY

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

When

110 J

of heat is added to a gaseous system, its internal energy increases by

40 J .

What is the amount of work done?

EASY

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

No work is done on the system, but

q

amount of heat is taken out from the system and given to the surroundings. Express the change in internal energy

(ΔU)

of this system and what type of wall does the system have?

EASY

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

When a system is taken from state $i$ to state $f$ along the path $i a f$ , it is found that $Q = 50 cal$ and $W = 20 cal$ . Along the path $i b f$ , $Q' = 36 cal$ . $W$ along the path $i b f$ is,

Question Image

HARD

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

The equation of state of $n$ moles of a non-ideal gas can be approximated by the equation $(p + \frac{n^{2} a}{V^{2}}) (V - n b) = n R T$ where, $a$ and, $b$ are constant characteristics of the gas. Which of the following can represent the equation of a quasi-static adiabatic for this gas (assume that, $C_{V}$ is the molar heat capacity at constant volume is independent of temperature)?

EASY

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

Five moles of an ideal monoatomic gas with an initial temperature of

150 ° C

expand and in the process absorb

1500 J

of heat and does

2500 J

of work. The final temperature of the gas in

° C

is (ideal gas constant,

R = 8.314 J K^{- 1} {mol}^{- 1}

	Column I		Column II
(i)	Adiabatic expansion	(a)	No work done
(ii)	Isobaric expansion	(b)	Constant internal energy
(iii)	Isothermal expansion	(c)	Increase in intemal energy
(iv)	Isochoric expansion	(d)	Decrease in internal energy

Match the following ? Column I Column II (i) Adiabatic expansion (a) No work done (ii) Isobaric expansion (b) Constant internal energy (iii) Isothermal expansion (c) Increase in intemal energy (iv) Isochoric expansion (d) Decrease in internal energy

Important Questions on Thermodynamics

Learn and Prepare for any exam you want !

Match the following ?

Column I Column II

(i) Adiabatic expansion (a) No work done

(ii) Isobaric expansion (b) Constant internal energy

(iii) Isothermal expansion (c) Increase in intemal energy

(iv) Isochoric expansion (d) Decrease in internal energy