Prove that diagonals of a rectangle are of equal length.

Important Questions on Quadrilaterals
Prove that diagonals of a square are equal and they bisect each other at right angles.
and are the midpoints of opposite sides and of parallelogram . Prove that is a parallelogram.

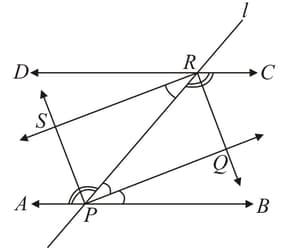
In the adjacent figure, and are two parallel lines which are intersected by transversal at points and respectively. Prove that the bisectors of the interior angles make a rectangle.
is an isosceles triangle in which , bisects the exterior angle and . Prove that
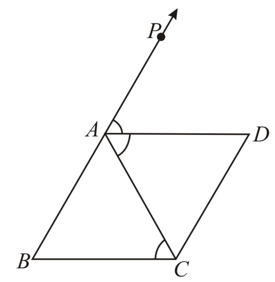
is an isosceles triangle in which , bisects the exterior angle and . Prove that quadrilateral is a parallelogram.

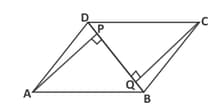
is a parallelogram and is one of its diagonals. and are perpendiculars on from the vertices and respectively. Prove that
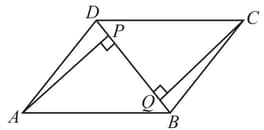
is a parallelogram and is one of its diagonals. and are perpendiculars on from the vertices and respectively. Prove that .
is a rectangle in which diagonal bisects both the angles and . Then prove that is a square.
