State Playfair’s axiom.
Important Questions on Lines and Angles
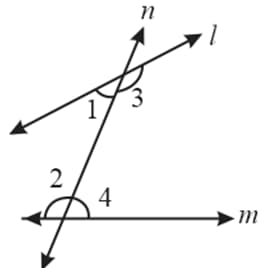
In the adjacent figure, a line falls on lines and such that the sum of the interior angle and is less than .On which side of the transversal will the line and line meet?
In how many points two distinct lines can intersect?
Two distinct lines cannot have more than one point in common.
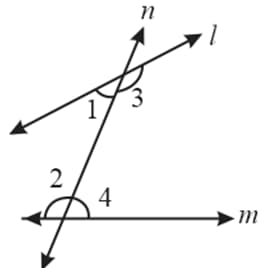
In the above figure, . If the lines and are produced indefinitely they will meet on that side on which .
Does Euclid fifth postulate imply the existence of parallel lines? Explain.
How many books are there in Euclid’s Elements ?
In the following figure, name two pairs of non-intersecting line segments.

In the adjoining figure, name :
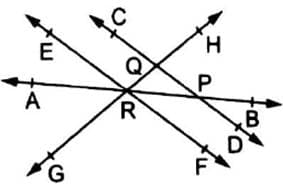
Two pairs of intersecting lines and their corresponding points of Intersection.
Is the following statement a direct consequence of Euclid's fifth postulate?
"There exists a pair of straight lines that are everywhere equidistant from one another."
Hint: Use play fairs axiom, which is equivalent to Euclid's fifth postulate.
Attempts to prove Euclid's fifth postulate using the other postulate and axioms led to the discovery of several other geometries.
If the diagonal of a square is ‘a’ units, what is the diagonal of the square, whose area is double that of the first square?

