Mathematics>Coordinate Geometry>The Straight Line>Basics of Straight Line

MEDIUM

Earn 100

The area of the triangle formed by two rays whose combined equation is $y = ∣ x ∣$ and the line $x + 2 y = 2$ is

50% studentsanswered this correctly

Important Questions on The Straight Line

HARD

Mathematics>Coordinate Geometry>The Straight Line>Basics of Straight Line

Let

O = (0, 0);

let

A

and

B

be points respectively on

x

-axis and

y

-axis such that

∠ O B A = 60 °

. Let

D

be a point in the first quadrant such that

O A D

is an equilateral triangle. Then the slope of

D B

is

MEDIUM

Mathematics>Coordinate Geometry>The Straight Line>Basics of Straight Line

M

and

N

are two points on the

x

axis and

y

axis respectively.

P (3, 2)

divides the line segment

M N

in the ratio

2 : 3

. Find the slope of the line

M N

.

EASY

Mathematics>Coordinate Geometry>The Straight Line>Basics of Straight Line

Find the equation of straight line whose slope is

\frac{10}{3}

and passes through the point

(0, 5)

.

EASY

Mathematics>Coordinate Geometry>The Straight Line>Basics of Straight Line

Find the equation of that straight line which passes through the points

(6, 0)

and whose slope is

\frac{7}{3}

.

EASY

Mathematics>Coordinate Geometry>The Straight Line>Basics of Straight Line

If the tangent at the point

P

on the circle

x^{2} + y^{2} + 6 x + 6 y = 2

meets

the

straight

line

5 x - 2 y + 6 = 0

at a point

Q

on the

Y

-axis, then the length of

P Q

is

HARD

Mathematics>Coordinate Geometry>The Straight Line>Basics of Straight Line

A triangle has vertices at

(6, 7), (2, - 9)

and

(- 4, 1)

. Find the slope of its medians.

MEDIUM

Mathematics>Coordinate Geometry>The Straight Line>Basics of Straight Line

The vertices of a

△ A B C

are

A (3, 8), B (- 1, 2)

and

C (6, - 6)

. Find slope of

B C

.

EASY

Mathematics>Coordinate Geometry>The Straight Line>Basics of Straight Line

The points

(0, \frac{8}{3}), (1, 3), (82, 30)

EASY

Mathematics>Coordinate Geometry>The Straight Line>Basics of Straight Line

The points

(0, \frac{8}{3}), (1, 3)

and

(82, 30)

MEDIUM

Mathematics>Coordinate Geometry>The Straight Line>Basics of Straight Line

If

α, β

are natural numbers such that

100^{α} - 199 β = (100) (100) + (99) (101) + (98) (102) + \dots . . + (1) (199),

then the slope of the line passing through

(α, β)

and origin is:

EASY

Mathematics>Coordinate Geometry>The Straight Line>Basics of Straight Line

Slope of a line is

3

and

y

intercept is

- 4

. Write the equation of a line.

EASY

Mathematics>Coordinate Geometry>The Straight Line>Basics of Straight Line

Find the y-intercept of the line joining two points

(1, 3)

and

(3, 5)

.

MEDIUM

Mathematics>Coordinate Geometry>The Straight Line>Basics of Straight Line

Slope of the straight line which is perpendicular to the straight line joining the points

(- 2, 6)

and

(4, 8)

is equal to:

EASY

Mathematics>Coordinate Geometry>The Straight Line>Basics of Straight Line

A line passes through the points

(8, 5)

and

(10, 6)

, then find the slope of the line.

MEDIUM

Mathematics>Coordinate Geometry>The Straight Line>Basics of Straight Line

Find the equation the straight line whose slope is

\frac{2}{3}

and passing through

(5, - 4)

.

EASY

Mathematics>Coordinate Geometry>The Straight Line>Basics of Straight Line

What is the slope of the line passing through the points $(5, 0)$ and $(3, 2)$ ? Write the equation of the line.

MEDIUM

Mathematics>Coordinate Geometry>The Straight Line>Basics of Straight Line

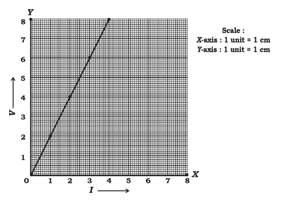
A student, while conducting an experiment on Ohm’s law, plotted the graph according to the given data. Find the slope of the line obtained.

$X$ -axis $I$	$1$	$2$	$3$	$4$
$Y$ -axis $V$	$2$	$4$	$6$	$8$

Question Image

MEDIUM

Mathematics>Coordinate Geometry>The Straight Line>Basics of Straight Line

A straight line with negative slope passing through

(1, 1)

cuts the

x

-axis at

A

and

y

-axis at

B

, where

O

is the origin. If

θ = ∠ O A B

, then the area of the triangle

O A B

is

EASY

Mathematics>Coordinate Geometry>The Straight Line>Basics of Straight Line

A line cuts off equal intercepts on the co-ordinate axes. The angle made by this line with the positive direction of X-axis is

MEDIUM

Mathematics>Coordinate Geometry>The Straight Line>Basics of Straight Line

A rectangle is inscribed in a circle with a diameter lying along the line

3 y = x + 7 .

If the two adjacent vertices of the rectangle are

(- 8, 5)

and

(6, 5),

then the area of the rectangle

(

in sq. units

)

is: