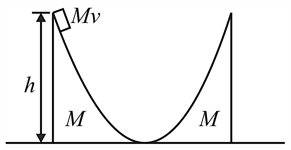
The inclined surfaces of two movable wedges of the same mass $M$ are smoothly conjugated with the horizontal plane (Fig.). A washer of mass $m$ slides down the left wedge from a height $h$.

To what maximum height will the washer rise along the right wedge? Friction should be neglected.

1.55. A symmetric block of mass $m_{1}$ with a notch of hemispherical shape of radius $r$

rests on a smooth horizontal surface near the wall (Fig.). A small washer of mass $m_2$ slides without friction from the initial position.

Find the maximum velocity of the block.

Determine the time dependences of the displacement $x_{\mathrm{wash} \mathrm{of} }$ of the washer and of its velocity $v_{\mathrm{wash}}$ relative to the table, starting from the instant $t_0$ and assuming

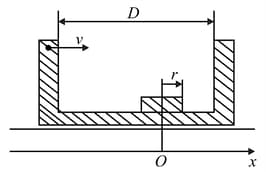
that all the impacts of the washer against the box are perfectly elastic. Plot the graphs $x_{\mathrm{wash}}\left(t\right)$ and $v_{\mathrm{wash}}\left(t\right)$ The friction between the box and the washer should be neglected.

A ring of mass $m$ connecting freely two identical thin hoops of mass $M$ each starts sliding down. The hoops move apart over a rough horizontal surface.

Determine the acceleration $a$ of the ring at the initial instant if $\angle A{O}_1{O}_2=\alpha$

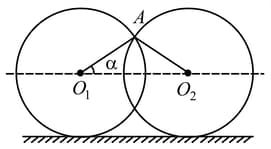
(Fig.), neglecting the friction between the ring and the hoops.

A flexible pipe of length $l$ connects two points $A$ and $B$ in space with an altitude difference $h$ (Fig.). A rope passed through the pipe is fixed at point $A$.

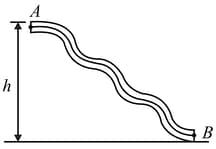
Determine the initial acceleration $a$ of the rope at the instant when it is released, neglecting the friction between the rope and the pipe walls.

A smooth washer impinges at a velocity $v$ on a group of three smooth identical blocks resting on a smooth horizontal surface as shown in Fig. The mass of each block is equal to the mass of the washer. The diameter of the washer and its height are equal to the edge of the block.

Determine the velocities of all the bodies after the impact.

Three small bodies with the mass ratio $3:4:5$ (the mass of the lightest body is $m$) are kept at three different points on the inner surface of a smooth hemispherical cup of radius $r$. The cup is fixed at its lowest point on a horizontal surface. At a certain instant, the bodies are released.

Determine the maximum amount of heat $Q$ that can be liberated in such a system. At what initial arrangement of the bodies will the amount of liberated heat be maximum? Assume that collisions are perfectly inelastic.