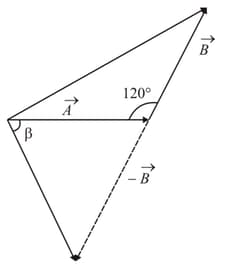
The maximum and minimum magnitudes of the resultant of two forces are and . Calculate the magnitude of individual forces.
Important Questions on Motion in a Plane
In an octagon of equal side, what is the sum of if,
Consider a frame that is made up of two thin massless rods and as shown in the figure. A vertical force of magnitude is applied at point of the frame.

Suppose the force is resolved parallel to the arms and of the frame. The magnitude of the resolved component along the arm is . The value of , to the nearest integer, is ________.
[Given : ]
Match List I with List II.
| List I | List II | ||
| (a) | (i) | 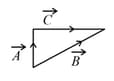 |
|
| (b) | (ii) | 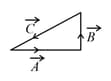 |
|
| (c) | (iii) | 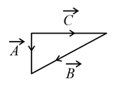 |
|
| (d) | (iv) | 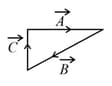 |
|
Choose the correct answer from the options given below:
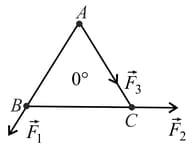
Figure shows three forces and acting along the sides of an equilateral triangle. If the total torque acting at point (centre of the triangle) is zero then the magnitude of is
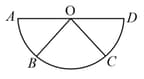
If are four points on a semi-circular arc with a centre at such that Then,
Polygon law of vector addition yields
In the light of the above statements, choose the most appropriate answer from the options given below.