The meeting point of the angle bisectors of a triangle is called the .
Important Questions on Geometry
Consider the following statements:
The orthocentre of a triangle always lies inside the triangle.
The centroid of a triangle always lies inside the triangle.
The orthocentre of a right-angled triangle lies on the triangle.
The centroid of a right-angled triangle lies on the triangle.
Which of the above statements are correct?
Consider the following statements:
1. The point of intersection of the perpendicular bisectors of the sides of a triangle may lie outside the triangle.
2. The point of intersection of the perpendiculars drawn from the vertices to the opposite sides of a triangle may lie on two sides.
Which of the above statements is/are correct?
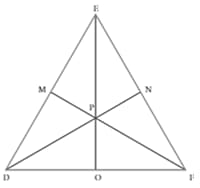
In are medians and point is the centroid. Find the following.
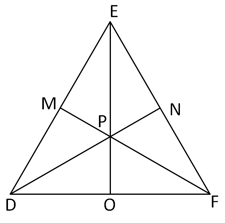
IF , then
In are medians and point is the centroid. Find the following.
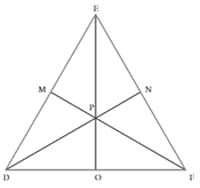
IF , then
Draw all three altitudes for the following triangles and explain how they are different:
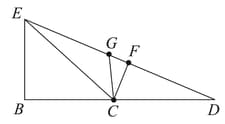
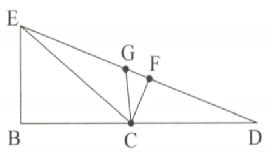
In the given figure, if is the midpoint of , name the median of .