Two blocks of masses $10 \mathrm{kg}$ and $30 \mathrm{kg}$ are placed on the same straight line with coordinates $\left(0,0\right)\mathrm{cm}$ and $\left(x,0\right)\mathrm{cm}$ respectively. The block of $10 \mathrm{kg}$ is moved on the same line through a distance of $6 \mathrm{cm}$ towards the other block. The distance through which the block of $30 \mathrm{kg}$ must be moved to keep the position of centre of mass of the system unchanged is

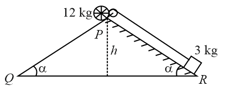
A rolling wheel of $12 \mathrm{kg}$ is on an inclined plane at position $P$ and connected to a mass of $3 \mathrm{kg}$ through a string of fixed length and pulley as shown in figure.
Consider $PR$ as friction free surface.
The velocity of centre of mass of the wheel when it reaches at the bottom $Q$ of the inclined plane $PQ$ will be $\frac{1}{2}\sqrt{xgh} \mathrm{m} {\mathrm{s}}^{-1}$. The value of $x$ (rounded off to the nearest integer) is _____.

Match List-I with List-II

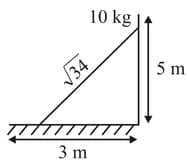
A $\sqrt{34} \mathrm{m}$ long ladder weighing $10 \mathrm{kg}$ leans on a frictionless wall. Its feet rest on the floor $3 \mathrm{m}$ away from the wall as shown in the figure. If $F_f$ and $F_w$ are the reaction forces of the floor and the wall, then ratio of $\frac{F_w}{F_f}$ will be :
(Use $g=10 \mathrm{m} {\mathrm{s}}^{-2}$.)

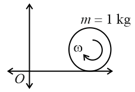
A spherical shell of $1 \mathrm{kg}$ mass and radius $R$ is rolling with angular speed $\omega$ on horizontal plane (as shown in figure). The magnitude of angular momentum of the shell about the origin $O$ is $\frac{a}{3}{R}^2\omega$. The value of $a$ will be