MEDIUM
Earn 100
Two moles of an ideal gas have undergone a cyclic process . If net heat exchange in the process is , then work done by the gas in the process is
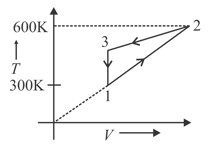

(a)
(b)
(c)
(d)none of these
50% studentsanswered this correctly
Important Questions on Thermodynamics
HARD
HARD
The equation of state of moles of a non-ideal gas can be approximated by the equation where, and, are constant characteristics of the gas. Which of the following can represent the equation of a quasi-static adiabatic for this gas (assume that, is the molar heat capacity at constant volume is independent of temperature)?
MEDIUM
Two moles of an ideal monoatomic gas occupies a volume at . The gas expands adiabatically to a volume . Calculate the final temperature of the gas and change in its internal energy.
HARD
| Column – 1 | Column – 2 | Column – 3 |
| (I) | (i) Isothermal | (P) 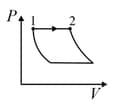 |
| (II) | (ii) Isochoric | (Q) 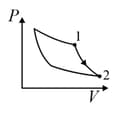 |
| (III) | (iii) Isobaric | (R)  |
| (IV) | (iv) Adiabatic | (S)  |
HARD
EASY
EASY
HARD
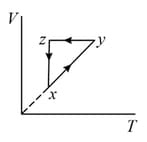
The - diagram that best describes this cycle is: (Diagrams are schematic and not to scale)
HARD

MEDIUM
EASY
HARD
EASY
MEDIUM
MEDIUM

MEDIUM
MEDIUM
EASY
EASY
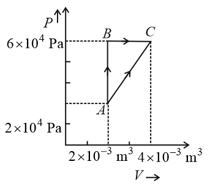
In process AB, of heat is added to the system and in process BC, of heat is added to the system. The heat absorbed by the system in the process AC will be:
MEDIUM
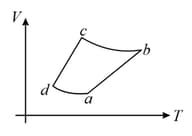
The corresponding P - V diagram for the process is (all figures are schematic and not drawn to scale) :

