EASY
Earn 100
What is the initial phase in Simple Harmonic Motion ?
Important Questions on Oscillations
HARD
MEDIUM
EASY
HARD
EASY
MEDIUM
EASY
MEDIUM
MEDIUM
The position co-ordinates of a particle moving in a coordinate system is given by
and
The speed of the particle is:
EASY
HARD
The motion of a mass on a spring, with spring constant is as shown in figure.
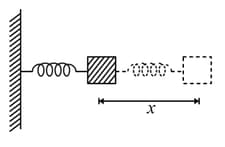
The equation of motion is given by, with .
Suppose that at time the position of mass is and velocity then its displacement can also be represented as where and are
EASY
MEDIUM
MEDIUM
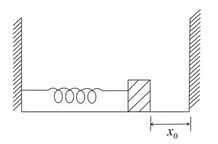
One end of a spring of force constant is fixed to a vertical wall and the other to a block of mass resting on a smooth horizontal surface. There is another wall at a distance , from the block. The spring is then compressed by and released. The time taken by the block to strike the other wall is
HARD
EASY
EASY
EASY
EASY
EASY

