Where does the orthocentre lie in the case of a right angled triangle?

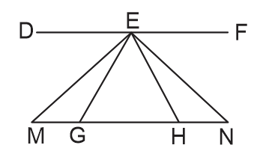
In the above figure, $DF$ is parallel to $MN$. $EGH$ is an isosceles triangle, where $EG=EH$ and $\angle GEH=50°$. If $EM$ and $EN$ are the bisectors of the $\angle DEG$ and $\angle EFH$, then

Show that $\angle GEM=\angle HEN$.

In an obtuse - angled triangle, the obtuse angled is $110°$. Find the angle made on its orthocentre.

The point of two external angle bisector for any side of a triangle and internal angle bisector of the angle opposite side chosen is called 

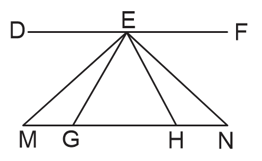
In the above figure, $DF$ is parallel to $MN$. $EGH$ is an isosceles triangle, where $EG=EH$ and $\angle GEH=50°$. If $EM$ and $EN$ are the bisectors of the $\angle DEG$ and $\angle EFH$, then

Show that $\angle DEM=\angle FEN$.

If the coordinates of the mid-points of the sides of a triangle are $\left(5,2\right), (3,3)$ and $\left(2,2\right)$. Find its centroid.

Where does the orthocentre lie in the case of an obtuse-angled triangle?

The circumcentre is defined as:

In $△ABC,$ the median $AD, BE$ and $CF$ passes through the point $G$. If $GF=4 \mathrm{cm}$ then, find $GC$.

The excentre is defined as:

Where does the orthocentre lie in the case of an acute-angled triangle?

In figure, point $G$ is the point of concurrence of the medians of $△PQR$. If $GT=2.5$, find the lengths of $PT$.

Find in how many points do the three medians of a triangle meet.

In $△ABC,$ the median $AD, BE$ and $CF$ passes through the point $G$. If $AD=7.5 \mathrm{cm},$ then find $GD$ (in $\mathrm{cm}$) (correct up to one decimal place)

The point of intersection of the perpendicular bisectors of the sides of a triangle is called: