Wien’s Displacement Law

Following graphs show the variation in the intensity of heat radiations by the black body and frequency at a fixed temperature. Choose the correct option.

Solar radiation emitted by sun corresponds to that emitted by the black body at a temperature of $6000 \mathrm{K}$. Maximum intensity is emitted at a wavelength of $4800$$\stackrel{\circ }{\mathrm{A}}$. If the sun was to cool down from $6000 \mathrm{K}$to $3000 \mathrm{K}$, then the peak intensity of emitted radiation would occur at a wavelength

Black holes in orbit around a normal star are detected from the earth due to the frictional heating of infalling gas into the black hole, which can reach temperatures greater than ${10}^6 \mathrm{K}$. Assuming that the infalling gas can be modelled as a blackbody radiator then the wavelength of maximum power lies

Assertion : Wien's displacement law fails for short wavelengths.

Reason : Intensity of radiations of very short wavelength is large.

The earth radiates in the infra-red region of the spectrum. The spectrum is correctly given by,

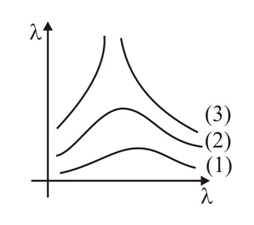
Three graphs marked as $1$, $2$, $3$ representing the variation of maximum emissive power and wavelength of radiation of the sun, a welding arc and a tungsten filament. Which of the following combination is correct

A solid body is heated upto very high temperatures. As we go on heating, its brightness increases and it appears white at the end. The sequene of the colour observed as the temperature of the body increases will be-

The spectral emissive power of a black body at a temperature of $6000 \mathrm{K}$ is maximum at ${\lambda }_{\mathrm{m}}=5000 \stackrel{\circ }{\mathrm{A}}.$ If the temperature is increased by $10\%,$ then the decrease in ${\lambda }_{\mathrm{m}}$ will be,

If maximum spectral emissivity at temperature $T_1 \mathrm{K}$ is at wavelength ${\lambda }_1,$ then the wavelength of maximum emissivity at temperature $T_2 \mathrm{K}$ will be-

If the temperature of a lamp is about $600 \mathrm{K},$ then the wavelength at which maximum emission takes place will be- (Wien's constant $b=3\times {10}^{-3} \mathrm{m} \mathrm{K}$)

After heating two pieces of iron, they are taken in dark room. One of them appears red and another appears blue, then-

Temperature of black body is $3000 \mathrm{K}.$ When black body cools, then change in wavelength $\mathrm{\Delta }\lambda =9$ micron corresponding to maximum energy density. Now temperature of black body is, 

The Wien's displacement law express relation between-

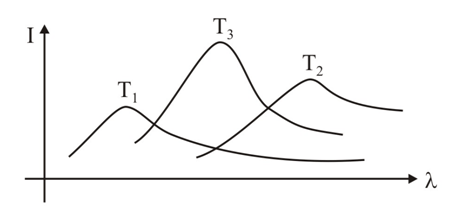
The plots of intensity vs. wavelength for three black bodies at temperatures $T_1, T_2$ and $T_3$ respectively are as shown. Their temperatures are such that-

At $T=200 \mathrm{K}$ a black body emits maximum energy at a wavelength of $14 \mu \mathrm{m}.$ Then at $T=1000 \mathrm{K}$ the body will emit maximum energy at a wavelength of-

The wavelength of maximum intensity for emitted radiation from a source is $11\times {10}^{-5} \mathrm{cm}$.  The temperature of this source is $n$ times the temperature of some other source for which the wavelength at maximum intensity is known to be $5.5\times {10}^{-5} \mathrm{cm}$. Find the value of $n$.

If at temperature $T_1=1000 \mathrm{K},$ the wavelength is $1.4\times {10}^{-6} \mathrm{m},$ then at what temperature the wavelength will be $2.8\times {10}^{-6} \mathrm{m}$

Let there be four articles having colours blue, red, black and white. When they are heated together and allowed to cool, which article cool at the earliest.

Assertion Blue star is at high temperature than red star.
Reason Wien's displacement law states that $T\propto \left(\frac{1}{{\lambda }_m}\right).$

When the temperature of a black body increases by $0.1\%$, the wavelength corresponding to maximum emission changes by $0.13 \mathrm{\mu m}$. The initial wavelength corresponding to maximum emission is,