Constraint Relations
Constraint Relations: Overview
This topic covers concepts such as Constraint Equations, String Constraint, Wedge Constraint, Constraint Equation in Inclined Planes, Constraint Equation in Wedges, Motion of Connected Bodies, and Motion on Inclined Plane.
Important Questions on Constraint Relations
The coefficient of static friction, , between block of mass and the table as shown in the figure is . What would be the maximum mass value of block so that, the two blocks do not move? The string and the pulley are assumed to be smooth and massless .
A block has been placed on an inclined plane with the slope angle block slides down the plane at constant speed. The coefficient of kinetic friction is equal to :
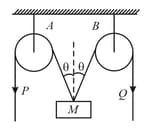
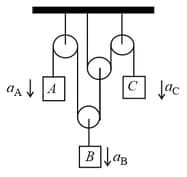
In the arrangement shown in the figure, the points and of the two inextensible strings move downwards with uniform speed . If the pulleys and are fixed, then the mass moves upwards with a speed
A light string passing over a smooth light pulley connects two blocks of masses m1 and m2 (vertically). If the acceleration of the system is , then the ratio of the masses is –
Two masses and tied to a string are hanging over a light frictionless pulley. What is the acceleration of the masses when left free to move? ( )
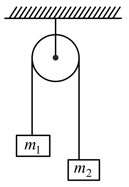
Two masses and tied to a string are hanging over a light frictionless pulley. What is the acceleration of the masses when left free to move? ( )

A particle starts sliding down a frictionless inclined plane. If is the distance travelled by it from time to , the ratio is
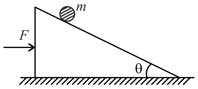
In the given figure, the wedge is acted upon by a constant horizontal force . The wedge is moving on a smooth horizontal surface. A ball of mass is at rest relative to the wedge. The ratio of forces exerted on by the wedge when is acting, and when is withdrawn, assuming no friction between the wedge and the ball, the ratio is equal to:
Determine the constraint equation which relates the accelerations of bodies and as shown:
Considering pulleys to be light & smooth and strings light. In the given below arrangement which is in equilibrium, mass is displaced further by and released find its acceleration just after it is released.
Two infinitely long rods are arranged on a plane making an angle with each other, as shown in the figure. The rod B starts to move with a uniform velocity in a direction perpendicular to rod Thus, the point of intersection moves with a horizontal velocity Which of the following statements is true ?

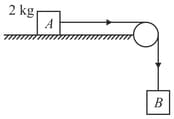
A block of mass is pulled along a horizontal frictionless surface by a rope of mass . If a force is applied at the free end of the rope, the force exerted by the rope on the block is
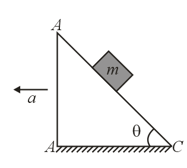
A block of mass resting on a wedge of angle as shown in the figure. The wedge is given an acceleration a towards left. What is the minimum value of a due to external agent so that the mass falls freely ?
A light string passing over a smooth light pulley connects two blocks of masses m1 and m2 (vertically). If the acceleration of the system is then the ratio of the masses is :
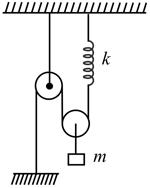
Two masses of and respectively are connected by a massless spring as shown in the below figure. A force of acts on the mass. At the instant shown the mass has acceleration . What is the acceleration of mass ?
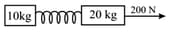
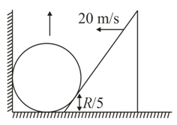
A sphere of radius is in contact with a wedge. The point of contact is from the ground as shown in the figure. Wedge is moving with velocity then the velocity of the sphere at this instant will be:
Three identical ballsare suspended on springs one below the other as shown in the figure. is a weightless thread. The balls are in equilibrium
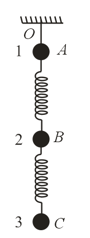
If the thread is cut, the system starts falling. Find the acceleration of all the balls at the initial instant
In the figure shown, two blocks one of mass and the other of mass are connected by light and inextensible string. Pulleys are light and frictionless. Choose the correct statement

Acceleration of pulleys and blocks are as shown in the figure. All pulleys strings are massless frictionless magnitude of and are