Amit M Agarwal Solutions for Chapter: Complex Numbers, Exercise 4: Work Book Exercise 4.4
Amit M Agarwal Mathematics Solutions for Exercise - Amit M Agarwal Solutions for Chapter: Complex Numbers, Exercise 4: Work Book Exercise 4.4
Attempt the free practice questions on Chapter 4: Complex Numbers, Exercise 4: Work Book Exercise 4.4 with hints and solutions to strengthen your understanding. Complete Study Pack for Engineering Entrances Objective Mathematics Vol 1 solutions are prepared by Experienced Embibe Experts.
Questions from Amit M Agarwal Solutions for Chapter: Complex Numbers, Exercise 4: Work Book Exercise 4.4 with Hints & Solutions
The straight line on the complex plane, has intercept on the imaginary axis equal to
If the complex number satisfies the condition then the least value of is equal to
for and then the centroid of the triangle formed by the points in the argand plane is given by
Let . If the origin and the non-real roots of form three vertices of an equilateral triangle in the argand plane, then is
If and are complex numbers representing the vertices of two triangles, such that and When is a complex number, then the two triangles
On the complex plane, and are similar and . If the points and denote the complex numbers and , then the complex numbers denoted by the point is given by
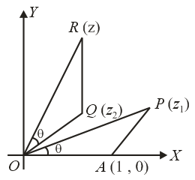
Intercept made by the circle on the real axis on complex plane, is
The equation of the radical axis of the two circles represented by the equations, and on the complex plane is
