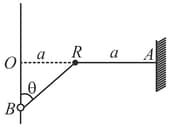
A small bead of mass is free to slide on a fixed smooth vertical wire, as indicated in the diagram. One end of a light elastic string, of unstretched length and force constant is attached to . The string passes through a smooth fixed ring and the other end of the string is attached to the fixed point , being horizontal. The point on the wire is at same horizontal level as , and .
(i) In the equilibrium position, find .
(ii) The bead is raised to a point of the wire above , where , and is released from rest. Find the speed of the bead as it passes , and find the greatest depth below of the bead in the subsequent motion.


Important Questions on Work, Energy and Power
A particle of mass approaches a region of force starting from The potential energy function in terms of distance from the origin is given by,
for
for where (positive constant)
(a) Derive the force and determine whether it is repulsive or attractive.
(b) With what velocity should the particle start at to cross over to other side of the origin.
(c) If the velocity of the particle at is towards the origin describe the motion.
A single conservative force acts on a particle that moves along the -axis. The potential energy is given by: where x is in meters. At the particle has a kinetic energy of .
(i) What is the mechanical energy of the system?
(ii) Make a plot of as a function of for and on the same graph draw the line that represents the mechanical energy of the system.
(iii) The least value of .
(iv) The greatest value of between which the particle can move.
(v) The maximum kinetic energy of the particle.
(vi) The value of at which it occurs.
(vii) Determine the equation for as a function of .
(viii) For what value of does
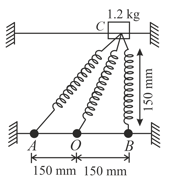
A collar may slide without friction along a fixed smooth horizontal rod. It is attached to three springs each of constant and undeformed length. Knowing that the collar is released from rest in the position shown. Determine the maximum velocity it will reach in its motion. [Here are fixed points.]
A block of mass is moved from rest on a smooth inclined plane of inclination by applying a constant force of parallel to the incline. The force acts for two seconds.
(a) Show that the work done by the applied force is not less than .
(b) Find the work done by the force of gravity in that two seconds if the work done by the applied force is .
(c) Find the kinetic energy of the block at the instant the force ceases to act in case (b). [Take ]
