A uniform cylinder of radius and mass is mounted to rotate freely about a horizontal axis that is parallel to and from the central longitudinal axis of the cylinder.
(a) What is the rotational inertia of the cylinder about the axis of rotation? (b) If the cylinder is released from rest with its central longitudinal axis at the same height as the axis about which the cylinder rotates, what is the angular speed of the cylinder as it passes through its lowest position?

Important Questions on Rotation
(c) At what angle is the tangential acceleration equal to ?
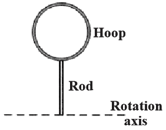
A uniform spherical shell of mass and radius can rotate about a vertical axis on frictionless bearings (In the figure shown below). A massless cord passes around the equator of the shell, over a pulley of rotational inertia and radius , and is attached to a small object of mass . There is no friction on the pulley's axle; the cord does not slip on the pulley. What is the speed of the object when it has fallen after being released from rest? Use energy considerations.
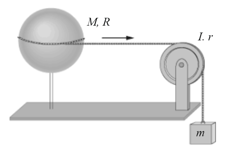
In the figure shown below a rigid assembly of a thin hoop (of mass and radius ) and a thin radial rod (of mass and length ). The assembly is upright, but if we give it a slight nudge, it will rotate around a horizontal axis in the plane of the rod and hoop, through the lower end of the rod. Assuming that the energy given to the assembly in such a nudge is negligible, what would be the assembly's angular speed about the rotation axis when it passes through the upside-down (inverted) orientation?