This diagram shows an electron tube. Electrons emitted from the cathode accelerate towards the anode and then Explain why the beam curves upwards between the plates pass into a uniform electric field created by two oppositely charged horizontal metal plates.

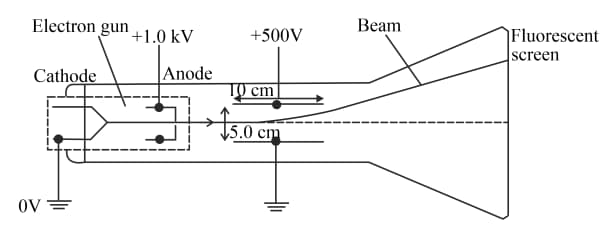
(b) Write down an equation relating the speed of the electrons v to the potential difference $V_{ac}$ between the anode and the cathode.

This diagram shows an electron tube. Electrons emitted from the cathode accelerate towards the anode and then explain why the beam curves upwards between the plates pass into a uniform electric field created by two oppositely charged horizontal metal plates.

(c) The deflection of the beam upwards can be cancelled by applying a suitable uniform magnetic field in the space between the parallel plates.

(i) State the direction of the magnetic field for this to happen.

This diagram shows an electron tube. Electrons emitted from the cathode accelerate towards the anode and then Explain why the beam curves upwards between the plates pass into a uniform electric field created by two oppositely charged horizontal metal plates.

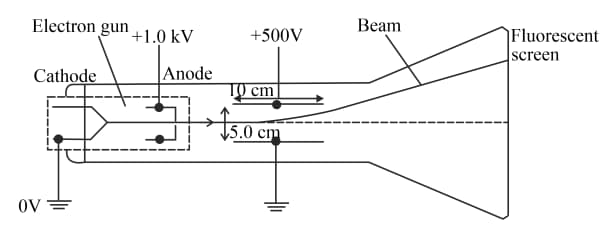
(c) The deflection of the beam upwards can be cancelled by applying a suitable uniform magnetic field in the space between the parallel plates.

(ii) Write down an equation relating the speed of the electrons $v$, the electric field strength $E$ that exists between the plates and the magnetic flux density $B$ needed to make the electrons pass undeflected between the plates.

This diagram shows an electron tube. Electrons emitted from the cathode accelerate towards the anode and then explain why the beam curves upwards between the plates pass into a uniform electric field created by two oppositely charged horizontal metal plates.

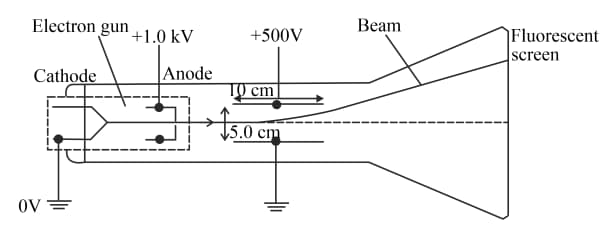
(c) The deflection of the beam upwards can be cancelled by applying a suitable uniform magnetic field in the space between the parallel plates.

(iii) Determine the value of $B$ required, using the apparatus shown in the diagram, given that for an electron the ratio$\frac{e}{m_e}=1.76\times {10}^{11} C k{g}^{-1}.$

Protons and helium nuclei from the Sun pass into the Earth's atmosphere above the poles, where the magnetic flux density is $6.0\times {10}^{-5}T.$ The particles are moving at a speed of $1.0\times {10}^{6}m{s}^{-1}$ at right angles to the magnetic field in this region. The magnetic field can be assumed to be uniform.

(a) Calculate the radius of the path of a proton as it passes above the Earth's pole.

Mass of a helium nucleus $=6.8\times {10}^{-27} kg$
Charge on a helium nucleus$=3.2\times {10}^{-19}C$

Mass of proton $=1.67\times {10}^{-27 }kg$

Charge on proton $=1.6\times {10}^{-19} C$

Protons and helium nuclei from the Sun pass into the Earth's atmosphere above the poles, where the magnetic flux density is$6.0\times {10}^{-5}T.$ The particles are moving at a speed of $1.0\times {10}^{6}m{s}^{-1}$ at right angles to the magnetic field in this region. The magnetic field can be assumed to be uniform.

(b) Sketch a diagram to show the deflection caused by the magnetic field to the paths of a proton and of a helium nucleus that both have the same initial velocity as they enter the magnetic field.

This diagram shows a thin slice of metal of thickness $t$ and width $d$. The metal slice is in a magnetic field of flux density $B$ and carries a current $I$, as shown.

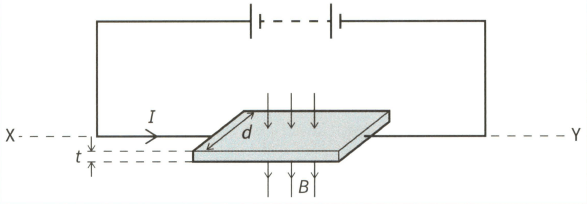
Copy the diagram and mark the slice that becomes negative because of the Hall effect.

This diagram shows a thin slice of metal of thickness $t$ and width $d$. The metal slice is in a magnetic field of flux density $B$ and carries a current $I$, as shown.

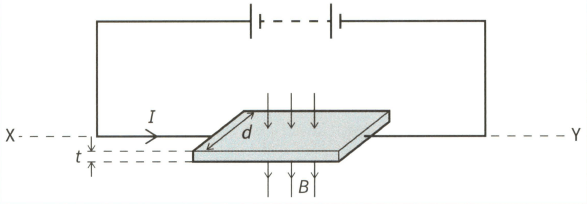
Copy the diagram and mark where a voltmeter needs to be placed to measure the Hall voltage.
 

This diagram shows a thin slice of metal of thickness $t$ and width $d$. The metal slice is in a magnetic field of flux density $B$ and carries a current $I$, as shown.

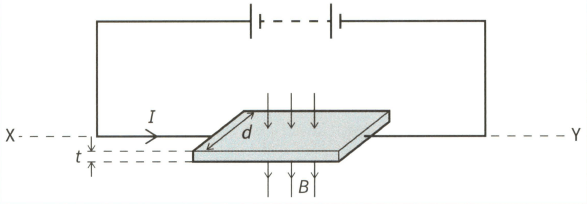
Derive an expression for the Hall voltage in terms of $I, B, t,$ the number density of the charge carriers $n$ in the metal and the charge on an electric iron.