Two wires between the same pair of supports are kept tight. The tensions in the wires are in the ratio $2 :1$ the radii are in the ratio $3 : 1$ and the densities are in the ratio $1 : 2$. Find the ratio of their fundamental frequencies.

A horizontal rod of length $40 \mathrm{cm}$ and uniform mass $1.2 \mathrm{kg}$ is supported by two identical wires as shown in figure. From the left end if a mass of $4.8 \mathrm{kg}$ be placed on the rod at $\alpha \mathrm{cm}$ so that the same tuning fork may excite the wire on left into its fundamental vibrations and that on right into its first overtone then find $\alpha$. $\left(\mathrm{g}=10 \mathrm{m} {\mathrm{s}}^{-2}\right)$.

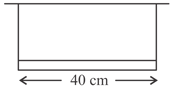
Figure shows a wire of aluminium of length $60 \mathrm{cm}$ joined to a steel wire of length $80 \mathrm{cm}$ and stretched between two given fixed supports. The tension produced is $40 \mathrm{N}$. The cross-sectional area of the steel wire is $1.0 {\mathrm{mm}}^2$ and that of the aluminium wire is $3.0 {\mathrm{mm}}^2.$ What could be the minimum frequency of a tuning fork which can produce standing waves in the system with their intersection as a node? The density of aluminium is $2.6 \mathrm{g} {\mathrm{cm}}^{-3}$ and that of steel is $7.8 \mathrm{g} {\mathrm{cm}}^{-3}$.

A string fixed at both ends has length $\mathrm{L}$ vibrates in its fundamental mode at a frequency $\nu$ and a maximum amplitude $A$.
(a) If the wavelength and the wave number are $\alpha L \mathrm{and} \frac{\mathrm{\beta \pi }}{\mathrm{L}}$.
(b) Taking origin at one end of the string and the $x-$axis along the string. Take the Y-axis along the direction of the displacement. Take $t=0$ at the instant when the middle point of the string passes through its mean position and is going towards the positive $y-$direction. If the equation describing the standing wave is $y=A\sin \left(\frac{\pi x}{L}\right)\sin \left(\gamma \pi \nu t\right)$.

Find $\alpha +\beta +\gamma$.

A $2$ m-long string fixed at both ends is set into vibrations in its first overtone. The wave speed on the string is $200 \mathrm{m} {\mathrm{s}}^{-1}$ and the amplitude is $0.5 \mathrm{cm}$
(a) If the frequency and wavelength are$\alpha \mathrm{m} \& \mathrm{\beta } \mathrm{Hz}$ .
(b)  The equation giving the displacement of different points as a function of time is $(0.5 \mathrm{cm})\sin \left[\left(\pi {\mathrm{m}}^{-1}\right)\mathrm{x}\right]\cos \left[\left(\gamma \pi {\mathrm{s}}^{-1}\right)\mathrm{t}\right]$. Assume X-axis along the string with the origin at one end and $t=0$ at the instant when the point $x=50 \mathrm{cm}$ has reached its maximum displacement.

Find $\alpha +\beta +\gamma$.

The equation for vibrating a string, fixed at both ends vibrating in its third harmonic, is given by $y=\left(0.4 \mathrm{cm}\right)\sin \left[\left(0.314 {\mathrm{cm}}^{-1}\right)\mathrm{x}\right]\cos \left[\left(600 \pi {\mathrm{s}}^{-1}\right)t\right]$ What is the speed of two travelling waves that can interfere to give this vibration in $m{s}^{-1}$?

The equation for a standing wave, produced on a fixed string at both ends, is

$y=\left(0.4\mathrm{cm}\right)\sin \left[\left(0.314{\mathrm{cm}}^{-1}\right)x\right]\cos \left[\left(600{\mathrm{\pi s}}^{-1}\right)t\right]$

What could be the smallest length of the string?

The figure shows a string stretched by a block going over a pulley. The string vibrates in its tenth harmonic in unison with a particular tuning fork. When a beaker containing water is brought under the block so that the block is completely dipped into the beaker, the string vibrates in its eleventh harmonic. The density of the material of the block is given as $k\times {10}^3 kg m^{-3}$. Find the value of $k.$