Magnetic Field on the Axis of a Circular Current Loop

Two concentric coils $X$ and $Y$ of radii $16 \mathrm{cm}$ and $10 \mathrm{cm}$ lie in the same vertical plane containing $N-S$ direction. $X$ has $20$ turns and carries $16 \mathrm{A}$. $Y$ has $25$ turns & carries $18 \mathrm{A}$. $X$ has current in anticlockwise direction. The magnitude of net magnetic field at their common centre is-

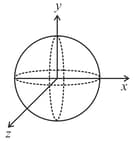
Three rings, each having equal radius R, are placed mutually perpendicular to each other and each having its centre at the origin of co-ordinate system. If current I is flowing through each ring then the magnitude of the magnetic field at the common centre is

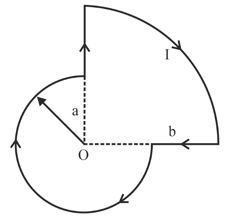
Find the magnetic induction at the origin in the figure shown.

Two circular coils A and B of radius $\frac{5}{\sqrt{2}}$ cm and 5 cm respectively current 5 Amp. and $\frac{5}{\sqrt{2}}$ Amp. respectively. The plane of B is perpendicular to plane of A their centres coincide. Find the magnetic field at the centre.

Find out the expression for the magnetic field at a point on the centre of a coil of radius carrying current I and having N number of turns.

Find out the magnetic field at axial point 'P' of solenoid shown in figure (where turn density $\text{'}n\text{'}$ and current through it is $I$)

The magnetic induction at the centre O is

The magnetic field at the centre of a current carrying loop of radius $0.1 \mathrm{m}$ is $5\sqrt{5}$ times that at a point along its axis. The distance of this point from the centre of the loop is(in $\mathrm{cm}$)

The magnetic field at the centre of a current carrying loop of radius 0.1 m is $5\sqrt{5}$ times that at a point along its axis. The distance of this point from the centre of the loop is

The magnitude of a magnetic field at the centre of a circular coil of radius $R$ , having $N$ turns and carrying a current $I$ can be doubled by changing

$\mathrm{A}$ conductor carrying current $i$ is bent in the form of concentric semicircles as shown in the figure. The magnetic field at the centre $O$ is

The magnetic field normal to the plane of $\mathrm{a}$ coil of $n$ turns and radius $r$ carrying $\mathrm{a}$ current $i$ is measured on the axis of the coil at $\mathrm{a}$ distance $h$ $\left(\mathrm{h}<<\mathrm{r}\right)$ from the centre of the coil. This is smaller than the field at the centre by the fraction

Two concentric coplanar circular loops of radia $r_1$ and $r_2$ cary currents $i_1$ and $i_2$ respectively, in opposite directions ($i_1$ clockwise and $i_2$ anticlockwise.) The magnetic induction at the centre of the loops is half of that due to $i_1$ alone at the centre. If $r_2=2r_1$, the value of $i_2/i_1=$

The surface charge density of a thin charged disc of radius $R$ is $\sigma .$ The value of the electric field at the centre of the disc is $\frac{\sigma }{2{\in }_0}$.

With respect to the field at the centre, the electric field along the axis at a distance $R$ from the centre of the disc :

Two identical wires $A$ and $B$, each of length $\text{'}𝑙\text{'}$, carry the same current $I$. Wire $A$ is bent into a circle of radius $R$ and wire $B$ is bent to form a square of side $\text{'}a\text{'}$. If $B_{\mathrm{A}}$ and $B_{\mathrm{B}}$ are the values of the magnetic field at the centres of the circle and square respectively, if the ratio$\frac{B_{\mathrm{A}}}{B_{\mathrm{B}}}$ is $\frac{{\mathrm{\pi }}^2}{ N\sqrt{2}}$ then the value of $N$ is :

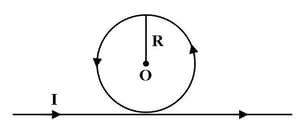
An infinitely long straight conductor is bent into the shape as shown below. It carries a current of $I$ ampere and the radius of the circular loop is $R$ metre. Then, the magnitude of magnetic induction at the centre of the circular loop is -

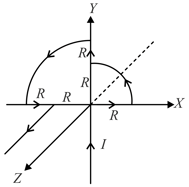
A current loop consists of two identical semicircular parts each of radius $R$, one lying in the x-y plane and the other in x-z plane. If the current in the loop is $i$, The resultant magnetic field due to the two semicircular parts at their common centre is

A straight conductor of length $l$ carrying a current $I,$ is bent in the form of a semicircle. The magnetic field (in tesla) at the centre of the semicircle is

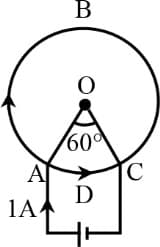
A cell is connected between the points $A$ and $C$ of a circular conductor $ABCD$ with $O$ as centre and angle $AOC=60°$. If $B_1$ and $B_2$ are the magnitudes of the magnetic fields at $O$ due to the currents in $ABC$ and $ADC$ respectively, then ratio $\frac{B_1}{B_2}$ is

Two circular current coils are concentric and their planes are mutually perpendicular and the magnetic field due to each coil is $B$, as shown in the figure: the net magnetic field at their common center will be