Angular Variables in Circular Motion
Angular Variables in Circular Motion: Overview
This topic covers concepts, such as, Linear Velocity in Circular Motion, Relation between Linear and Angular Velocity, Tangential Acceleration & Relation between Angular Acceleration and Tangential Acceleration etc.
Important Questions on Angular Variables in Circular Motion
Two particles of mass M and m are moving in a circle of radii R and r. If their time – periods are same, what will be the ratio of their linear velocities?
What is the linear velocity if the angular velocity vector is and position vector is ?
A body is whirled in a horizontal circle of radius 20 cm. It has an angular velocity of What is its linear velocity at any point on circular path
When a body moves with a constant speed along a circle
Spotlight rotates in a horizontal plane with a constant angular velocity of . The spot of light moves along the wall at a distance of . The velocity of the spot when (see. fig.) is

A solid body rotates an angle about a stationary axis according to the law . What is the mean value of angular velocity (in )over the time interval between and the time when the body comes to rest?
The angular displacement of a particle, rotating with constant angular acceleration is is measured in radian and in second. The angular acceleration of the particle is,
How much distance is covered by the tip of a minute hand of length 5 cm when it moves from 3 to 6
Determine the angular speed of a turntable in
A rod of length is pivoted at one end and is rotated with a uniform angular velocity, in a horizontal plane. Let and be the tensions at points and , away from the pivoted ends.
A particle when moves in a circle with a uniform speed:
A ring of radius rolls without slipping on a rough horizontal surface with a constant velocity. The radius of curvature at the highest point of the path followed by any particle on the ring :
A particle is moving in a circle of radius with a uniform speed . is the centre of the circle and is a diameter. When passing through the angular velocity of about and are in the ratio
A particle is moving in a circle with constant tangential acceleration. Which one of the following is constant?
A point moves along a circle such that distance travelled [where ] and is time in second. Find the total acceleration of the point at the moment when it covered the fraction of the circle after the beginning of the motion to the closest integer. [Take ]
One end of a string having length attached to the a ball of mass . The ball is rotated on a horizontal circular path about vertical axis. The maximum tension that the string can bear is . If maximum value of angular velocity of ball is N then what is the value of ?
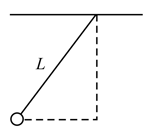
A body of mass is attached to one end of a wire of length The maximum angular speed ( in rad ) with which it can be rotated about its other end in space station is (Breaking stress of wire and area of cross section of the wire is :
The angle turned by a body undergoing circular motion depends on time as , Then the angular acceleration of the body is :
The angular speed of a car increases from to in . What is the angular acceleration of the car?
An object is moving around a circular path of radius with speed of . The value of tangential acceleration in Uniform circular motion is
