Parallelogram, Rhombus and Trapezium

If the side of a rhombus is $10 \mathrm{cm}$ and one diagonal is $16 \mathrm{cm}$, the area of the rhombus is

The ratio of semiperimeter of a parallelogram to its longer side is same as the ratio of its longer side to its shorter side. If the shorter side measures $2 \mathrm{cm}$, find the maximum possible area of the parallelogram.

In the adjoining figure, a parallelogram ABCD is shown. $\mathrm{AB}=24 \mathrm{cm}$ and $\mathrm{AO}=\mathrm{BO}=13 \mathrm{cm}$. Find BC.

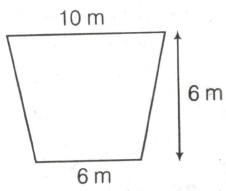
In the adjoining figure, the cross-section of a swimming pool is shown. If the length of the swimming pool is $120 \mathrm{m}$, then the amount of water it can hold is :

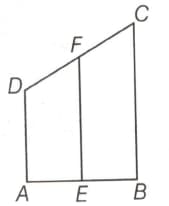
ABCD is a trapezium, in which $\mathrm{AD}\Vert \mathrm{BC},\mathrm{E}$ and F are the mid-points of AB and CD respectively, then EF is :

$\mathrm{ABCD}$ is a quadrilateral $\mathrm{AC}=19 \mathrm{cm}$. The lengths of perpendiculars from$\mathrm{B}$ and $\mathrm{D}$ on$\mathrm{AC} \text{are} 5\mathrm{cm}$ and$7 \mathrm{cm}$ respectively. Then, the area of$\mathrm{ABCD}$$\left(\text{ in }{\mathrm{cm}}^2\right)$) is :

The area of a hexagon whose one side is $4 \mathrm{m}$,is :

The cross-section of a canal is in the shape of a trapezium and the area of cross section is $360{ \mathrm{m}}^2$. If the canal is $12 \mathrm{m}$ wide at the top and $8 \mathrm{m}$ wide at the bottom the depth of the canal is :

The area of a trapezium is  $441{ \mathrm{cm}}^2$ and the ratio of parallel sides is $5: 9.$ Also the perpendicular distance between them is $21 \mathrm{cm}$, the longer of parallel sides is :

$\mathrm{A} \mathrm{B} \mathrm{C} \mathrm{D}$ is a trapezium in which$\mathrm{A} \mathrm{B}\left|\right| \mathrm{C} \mathrm{D}$ and $\mathrm{AB}=2\mathrm{CD}$. If its diagonals intersect each other at $\mathrm{O}$, then ratio of areas of triangles $\mathrm{AOB}$and $\mathrm{COD}$is :

The lengths of two parallel sides of a trapezium are $30 \mathrm{cm}$ and $50 \mathrm{cm}$ and its height is $16 \mathrm{cm}$. Its area is :

The ratio of the lengths of the diagonal of a rhombus is $2: 5.$ Then, the ratio of the area of the rhombus to the square of the shorter diagonal.

If the perimeter of a rhombus is $4$p and lengths of its diagonals are a and b, then its area is :

In the adjoining figure, the ratio of the areas of the parallelogram $\mathrm{ABCD}$and that of triangle  $\mathrm{ABN}$is :

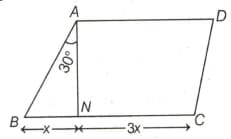
The two adjacent sides of a parallelogram are $25 \mathrm{cm}$ and $40 \mathrm{cm}$ respectively. The altitude drawn on the longer side is $18 \mathrm{cm}$, then the area of the parallelogram is :

The distance of a $24 \mathrm{cm}$ long side of a parallelogram from the opposite side is $22 \mathrm{cm}$. The area of the parallelogram is :

A parallelogram has sides $30 \mathrm{cm}$ and $20 \mathrm{cm}$ and one of its diagonal is $40 \mathrm{cm}$ long. Then its area is :

Area of a rhombus is $256 c{m}^2$. One of the diagonal is half of the other diagonal. The sum of the diagonals is _____.